“In science, one plus one is always two; in art it can also be three or more.” This quotation from the artist Josef Albers appears early on in Beautiful Geometry, and it makes an excellent motto for this slyly humorous and – yes – beautiful book about the most visual branch of mathematics. A collaboration between a historian of mathematics, Eli Maor, and a mathematically-inspired artist, Eugen Jost, the book contains 51 short essays about different topics in geometry, each of them accompanied by at least one full-colour artwork.

Throughout the book, these vibrant illustrations (a mix of computer-generated images and acrylic on canvas) function both as stand-alone artworks and as demonstrations of mathematical concepts – albeit with a dash of artistic licence from Jost, who is clearly a fan of Albers’ views on the sum of 1 + 1.

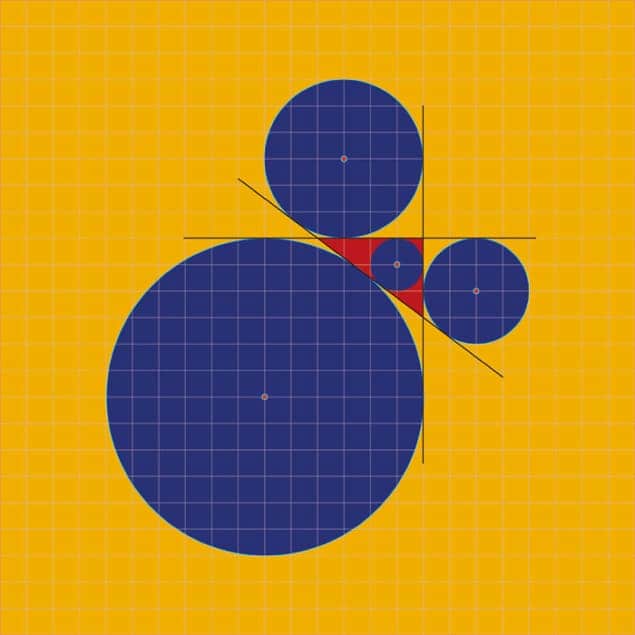
The journey through geometry begins with the Pythagoreans, the cult-like group in ancient Greece whose motto “Number rules the universe” reflected their belief in a mathematically ordered world. Their leader, Pythagorus, did not discover the theorem that bears his name, but he may have been the first to prove it, and his followers certainly used it to explore related geometric concepts. One such concept appears in Jost’s artwork “The (3,4,5) Triangle and Its Four Circles” (see image 1, above). At the heart of this image is a right triangle inscribed with an “incircle” that is tangent to all three sides. Outside the triangle are three “excircles”, each of which is tangent to one side of the triangle and to the continuations of the other two sides. The fact that the lengths of this triangle’s three sides form a “Pythagorean triple” (a trio of positive integers a, b and c for which a² + b² = c²) has a curious consequence: it means that the radii of the incircles and excircles must also be integers. This property, Maor notes, leads to some interesting relationships between the radii of the four circles; for example, the product of the radii of the smaller excircles r_a and r_b is equal to the area of the triangle, ab/2.

Such relationships delighted the Pythagoreans, but later developments in mathematics were less to their liking. As Maor explains, the discovery that √2 cannot be expressed as the ratio of two positive integers “brought about a serious intellectual crisis” among the group’s members.

Of course, knowledge of these new “irrational” numbers also brought about advances in geometry, including studies of the “golden ratio” that crops up in so many areas of art and nature (see image 3, above). And even the Pythagoreans might have been pleased with a few of the modern pieces of geometry that appear later in the book. Another image (number 2, above), for example, illustrates a theorem known as “Steiner’s porism”, which concerns the behaviour of chains of non-concentric circles. Its co-discoverer, Jakob Steiner, helped to revive “classical” geometry in the early 19th century and, despite its antiquity, this well-studied discipline turned out to have some new tricks up its sleeve.

A good example is Morley’s Theorem (see image 4, right), which involves angle trisection but could otherwise have come straight out of Euclid’s Elements. Even in classical geometry, Maor concludes, “surprises may still be awaiting us around the corner – or perhaps around the vertex!”