Polynomials are mathematical expressions involving a sum of powers in one or more variables multiplied by coefficients. Random polynomials are simply polynomials with random coefficients that have a Gaussian or bell-shaped probability distribution. Solving the roots of random polynomials is an important field in theoretical physics and although such polynomials have been extensively studied, no one has ever seen what these expressions might actually look like. Now, Castin and colleagues have shown that the location of “vortices” in a rotating 2D Bose-Einstein gas could be used to physically represent the roots of a polynomial.

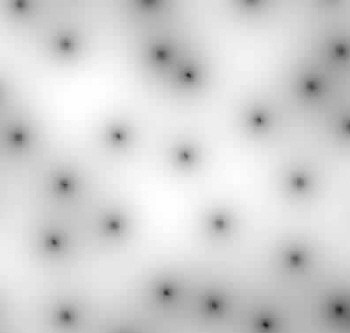
A Bose-Einstein condensate (BEC) is an ultra-cold cloud of gas atoms that are all in the same quantum state, and can therefore be described by the same wavefunction. Quantum vortices can form in these condensates if they are rapidly rotated. According to Castin and co-workers, the wavefunction of a rotating condensate formed with non-interacting atoms can be described by a random polynomial.

A vortex “sits” at each location where the wavefunction “vanishes”, and it can be associated with a root of the polynomial. Each root is a complex number with a real and imaginary part, which can be viewed as the two spatial coordinates of the vortex. The roots interact with each other and this is represented by the vortices repelling each other.

The team says that the fictitious BEC formed by the roots is interesting in this context because it is a very rare example of an exactly solvable many-body problem in physics. “The mathematical theory of random polynomials and random matrices has already found many applications in physics,” says team member Jean Dalibard. “Indeed the Hamiltonian of a complex or chaotic system can often be viewed as such a matrix.”

So how did the France-Italy team find the unlikely connection between random polynomial theory and BECs? “We were interested in the case of interacting atoms in which the roots of the polynomials — that is the location of the quantized vortices — form a regular array,” explains Dalibard. “To our surprise we noticed that even for strictly non-interacting atoms, a local order of the vortex distribution remained.” This is exactly what happens in mathematical random polynomials — although the coefficients of the polynomial are independent, the root distribution still exhibits some correlations.

The team says there is no fundamental barrier to why the experiment could not be performed in the near future. “Indeed, current experimental conditions are already less than an order of magnitude away from required conditions,” says Dalibard.