Scientists in the US have solved a problem in fluid dynamics that dates back more than 100 years, namely how to model precisely what happens when a layer of air or water detaches itself from a moving object. They have developed a theory to predict exactly where such separation should occur and confirmed these predictions experimentally, suggesting that vehicle manufacturers in the future might build cars that continually change shape very slightly in order to minimize drag.
When a solid object moves through a fluid, either liquid or gas, any variation in acceleration of the air over different parts of the object will create pressure distributions that affect how the air flows in the boundary layer immediately surrounding the object. A large enough pressure gradient can decelerate this boundary layer sufficiently so that it stagnates and then detaches itself from the object leading edge of the object — where the air hits the object full on.
According to Bernoulli’s principle, the lower velocity of the detached air layer will increase its pressure, so when the layer detaches itself from the leading edge of a moving car it will increase the pressure over the front of the vehicle and so oppose its forward motion (known as increasing drag). As regards to a moving aeroplane, the extra pressure over the wings will lead to a loss of lift, forcing the aircraft to lose altitude.
German physicist Ludwig Prandtl derived the mathematics of this flow separation in 1904. But his equations were restricted to steady two-dimensional flows — flows that move around objects with a constant velocity. As a result, his method cannot describe many real-world scenarios, such as cars accelerating or decelerating, or planes taking off and landing.
Success with dynamical systems theory
In 2004 George Haller at the Massachusetts Institute of Technology (MIT) and colleagues described mathematically how unsteady flow separation could occur in two dimensions, and his group has now extended this analysis to three dimensions ( Phys. Fluids 20 097101). Whereas Prandtl used traditional fluid mechanics to describe the state of a fluid at particular instances of time in terms of macroscopic quantities such as velocity, Haller instead applies a branch of applied mathematics known as dynamical systems theory to chart the evolution of individual parcels of fluid. This allows Haller to identify the exact location of structures within the fluid that guide the parcels away from the solid surface in question.
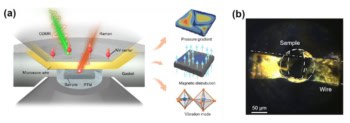
This theory has been at least partially validated by a group led by one of Haller’s colleagues at MIT, Thomas Peacock. Peacock and co-workers filled an acrylic 40 cm-long tank with the liquid glycerol, sandwiching it between layers of electric coolant below and vegetable oil above. They then set the fluid in motion by placing a tube in the middle of the tank and then using a motor to rotate the tube and move it from one side of the tank to the other.
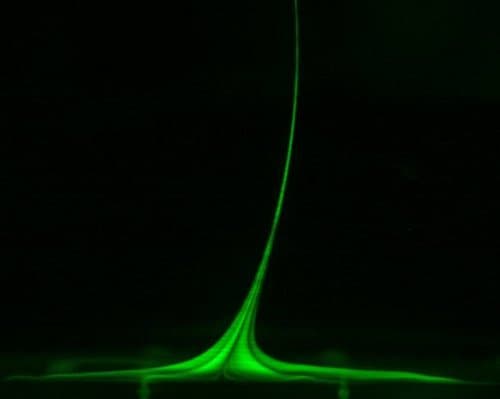
By inserting dye into the tank and then watching how this dye dispersed by illuminating it with a laser beam (which caused it to fluoresce), Peacock’s team were able to locate exactly where the glycerol separated from one of the side walls of the tank. This location matched very closely that predicted using Haller’s 2D theory when simulating the conditions within the tank, and showed, surprisingly, that even within a highly chaotic flow this location is fixed ( J. of Fluid Mech. 611 1).
Adjusting the shape of your car
According to Peacock this work could ultimately be used to improve vehicle performance by allowing a series of sensors and actuators to be distributed across the surface of a vehicle at those points where the theory predicts separation of the boundary layer should occur. Information about the air flow across the sensors would be used to make tiny adjustments to the shape of the vehicle body so as to delay or prevent this separation.
However, Peacock points out that this would only make sense if the energy saved more than made up for the extra cost and weight imposed by the high-tech gadgetry. He also notes that the theory in its present form cannot account for certain types of flow that were investigated in the tank — such as those due to disturbances with randomly varying periods of oscillation — but adds that Haller is working on extensions to the theory in order to address this. Peacock and colleagues also plan to test Heller’s 3D theory.