Hofstadter’s butterfly – a stunning fractal pattern that describes the behaviour of electrons in a magnetic field – has been measured experimentally for the first time. The breakthrough has been made by three research groups that looked at the electronic properties of graphene placed on a boron-nitride surface. As well as confirming a theoretical prediction made nearly 40 years ago, the results could find use in electronic and optoelectronic devices.
The concept of Hofstadter’s butterfly dates back to 1976 when the American physicist Douglas Hofstadter – best known for his Pulitzer-prize-winning book Gödel, Escher, Bach – calculated the energy levels of electrons exposed to a magnetic field in a 2D lattice. He treated the electrons as idealized “Bloch electrons”, which means they do not interact with one another and move in a periodic electric potential commensurate with the lattice. This approach seemed sensible as it had proved a good way of describing the electronic properties of many metals and semiconductors.
Unfortunately, Hofstadter encountered a difficulty when considering electrons exposed to a magnetic field while moving in a 2D lattice. The problem lies in the fact that electrons respond to an applied magnetic field by swimming in circles at the cyclotron frequency. However, the orbital motion of the electrons is quantized in terms of a frequency that is defined by the properties of the crystal lattice. The presence of these two, often incommensurate, frequencies means that Hofstadter’s calculation of electron energy levels yielded some bizarre results.
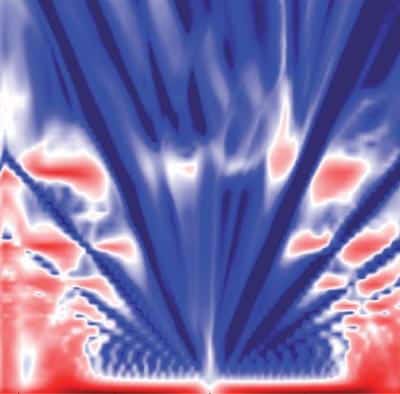
Hofstadter tried to make sense of this difficulty by plotting the wavefunction of the electron versus a parameter related to the ratio of the two frequencies. The plot produced a stunning fractal pattern that looks like a butterfly. But since Hofstadter’s prediction, physicists have struggled to observe his butterfly in the lab. The main problem is that the crystal frequencies of conventional lattices – where atoms are separated by less than a nanometre – are relatively high, which means that unfeasibly strong magnetic fields way beyond current capabilities would be needed to see the butterfly pattern. Physicists have also tried looking for the Hofstadter butterfly in artificial lattices with separations of hundreds of nanometres, which would require much smaller magnetic fields, but the effect is washed out by disorder in the system.
2D superlattices
Now, however, three research groups have got round this problem by combining two conventional 2D lattices to create superlattices with periodicities on the order of tens of nanometres. One team involved physicists at Columbia University, the City College of New York, the University of Central Florida – all in the US – and Tohoku University and the National Institute for Material Sciences in Japan. The second group included researchers at the universities of Manchester and Lancaster in the UK, France’s National Lab for High Magnetic Fields in Grenoble and the Institute of Materials Science in Madrid, Spain. The third group involved researchers from the Massachusetts Institute of Technology (MIT) who teamed up with the same Japanese researchers involved in the Columbia team.
What the teams did was place graphene – a layer of carbon just one atom thick – on an extremely flat surface of a boron-nitride crystal. As both materials have similar hexagonal structures, the researchers observed “Moiré patterns”, which are regular patterns created whenever two similar 2D lattices are overlaid. (The Manchester and MIT groups used single-layer graphene, while the Columbia-led experiment involved bilayer graphene.) By tweaking the relative orientation of the two lattices, the teams were able to create superlattices with appropriate spacing.
The teams then determined the energy spectrum of their superlattice by measuring its electrical conductivity in strong magnetic fields – up to about 35 T at Columbia, 17 T at Manchester and 43 T at MIT. When they plotted electron density (an observable property of the wavefunction) versus magnetic field strength, the teams saw the long-sought-after Hofstadter butterflies.
‘Rare and tremendously exciting’
“The opportunity to confirm a 40-year-old prediction in physics that lies at the core of most of our understanding of low-dimensional material systems is rare, and tremendously exciting,” says Cory Dean of City College of New York. “Our confirmation of this fractal structure opens the door for new studies of the interplay between complexity at the atomic level in physical systems and the emergence of new phenomenon arising from complexity.”
As well as confirming an important theoretical prediction, the studies provide further verification that the electronic properties of graphene can be controlled by placing the material next to another crystalline structure. This could prove useful because although graphene has several technologically useful properties – such as being brilliant at conducting electricity – it lacks key features, notably an electronic band gap, which would make it useful for creating practical transistors or optical detectors.
New materials
The Hofstadter butterfly observation is the latest in a long line of fascinating new findings involving graphene, which was first isolated by Andre Geim and Kostya Novoselov from the University of Manchester in 2004. “Of course, it is nice to catch the beautiful ‘butterfly’ whose elusiveness tormented physicists for generations,” says Geim, “[but] more importantly, this work shows that we are now able to build up a principally new kind of material by stacking individual atomic planes in a desired sequence.”
Geim’s colleague Roman Gorbachev even thinks such superlattices could have practical applications. “Such artificial crystals would have been science fiction a few years ago [but] now they are reality in our lab. One day you might find these structures in your gadgets.”
The Columbia and Manchester experiments are described in Nature (here and here) and a preprint of the MIT result is on arXiv.
- Physics World learns how to make graphene at the University of Manchester