There is a surprising lack of symmetry in Maxwell’s equations of electromagnetism. Electric fields are created by electric charges, while magnetic fields are produced by the movement of electric charges. Magnetic charges are completely absent from the theory, not because Maxwell forgot to put them in, but because isolated magnetic charges do not seem to exist in the real world.
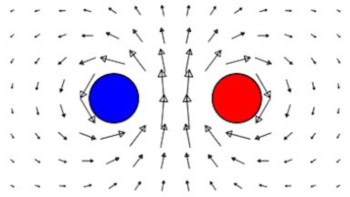
In 1931 Paul Dirac showed that if a magnetic charge exists, it has to be quantized in units of h/e,where h is Planck’s constant and is the charge on the electron. Now an experiment in Japan has found evidence for an effective magnetic charge – also known as a magnetic or Dirac monopole – in the abstract momentum space that is routinely used by condensed-matter physicists to analyse the properties of crystals.
The results, which rely on theoretical work by Zhong Fang of the National Institute of Advanced Industrial Science and Technology in Tsukuba, suggest that monopoles can have direct physical consequences in systems as common as a ferromagnet (Z Fang et al. 2003 Science 302 92).
We should stress that these are “effective” monopoles. Although they exist naturally in the ground state of the crystal, they have no meaning outside it. The coupling of electrons to these momentum-space monopoles is mathematically similar to their coupling to the real-space magnetic monopoles that have long been sought by particle physicists.
In the January issue of Physics World Allan MacDonald and Qian Niu in the Department of Physics at the University of Texas in the US describe this work in more detail.