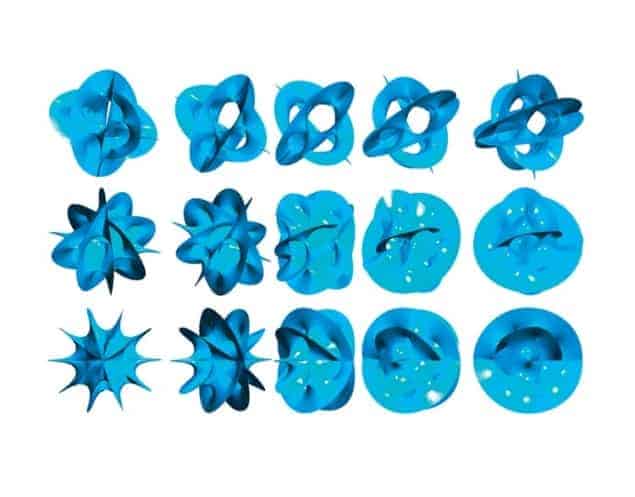
Calabi–Yau spaces have become the poster child for string theory. These baroquely curved shapes adorn book jackets and conference notices galore, and you can even buy a plum-size model of one from the website of sculptor Bathsheba Grossman. But for most people – even most physicists, I suspect – such higher-dimensional origami is simply an object of awe. In part, this is because books and magazine articles about string theory for the general public gloss over what these shapes actually are. As a result, Calabi–Yau spaces have become something to gawk at, but never really grasp – rather like string theory in general. It has fallen to eminent mathematician Shing-Tung Yau himself, working with science writer Steve Nadis, to try to demystify them. Although their book The Shape of Inner Space is very uneven, it takes a big step towards making Calabi–Yaus more than so much eye candy.

Like the frame of a harp, the shape of space determines how the microscopic strings of string theory vibrate – giving rise, so the theory goes, to all the elementary particles and forces of nature. In the standard formulation of the theory, in order for strings to reproduce the observed properties of those particles and forces, space must have six unseen dimensions in addition to the three visible ones, and those dimensions must be finite in extent and crumpled into a Calabi–Yau shape. Basically, Calabi–Yaus are finite spaces with a high degree of symmetry. Not only do they satisfy the equations of general relativity for a vacuum, they also have a subtle geometric symmetry that is related to the physics concept of supersymmetry.

Such spaces were first conjectured by Eugenio Calabi in 1953, and Yau’s 1976 proof that they can exist was one of the major achievements that won him a share of both the 1982 Fields Medal and this year’s Wolf Prize in Mathematics. But the book covers the full range of Yau’s work and the broader research programmes to which it contributed, such as the positive-energy conjecture in general relativity (confirming that space–time is stable), the Poincaré conjecture and mirror symmetry. Not bad for someone who once failed a primary-school mathematics entrance exam and briefly dropped out of school altogether! And although the book never forgets to remind the reader how (justifiably) proud Yau is of his achievements, it also gives credit to others, including some (such as Alexander Givental from University of California, Berkeley) with whom he has had priority disputes. It also acknowledges that luck and good timing play a big role in individual accomplishment.

Along the way, the book introduces readers to concepts seldom seen in material intended for the general public, such as parallel transport, holonomy, geometric flow and the ways that topology constrains geometry and vice versa. Above all, it is fascinating to see the story of string theory told from a mathematician’s point of view rather that of than a physicist. The book argues that the theory mended a schism that had opened up between the two disciplines. However, it is frank about the problems that string theorists have needed to confront, such as the question of which – if any – Calabi–Yau shapes are dynamically stable (the “moduli stabilization” problem). In an interesting take on the notorious controversy over the “landscape” of string theory, which calls into question whether string theory is capable even in principle of making firm predictions, Yau suggests that the problem may lie in an incomplete understanding of Calabi–Yaus. In fact, for all the attention paid to these shapes, they are not the only form that the extra dimensions of string theory might take.

The book is worth picking up just for the chapter that fleshes out the definition of Calabi–Yau spaces. But be warned: it does not make for light reading, and although it may be intended for a general readership, I doubt it will achieve this aim. Large chunks of it seem written for other mathematicians and physicists, for whom the explanations will come as a relief from the deadly theorem-proof-theorem-proof style of most mathematics texts. Beyond these circles, most readers’ heads are going to hurt.

One particular failing is that the book does not clarify when a word can be understood colloquially and when it has a specific technical meaning. Non-expert readers are not going to realize that “the Gauss curvature of a sphere” is not the same as “the total Gauss curvature of a sphere” and will therefore wonder what they have missed when told that one has a value of 1 (for a unit sphere) and the other 4p. What is more, two chapters pass between the definition of Gauss curvature and further discussion of the concept. This is far too long an interval for most readers to remember what it means.

The book gives technical definitions that may be important to maintain rigour but will come across to non-specialist readers as unmotivated and impossible to parse. Even the introductory chapters take it for granted that readers already have an interest in geometry. Ironically, the later chapters are easier to absorb than the early ones, as though the authors realized in the process of writing the book that they had to tone it down. A chapter about vacuum decay seems positively fluffy compared with the dense earlier material.

It is also a little surprising that, after introducing Calabi–Yaus, the book does not venture to describe what they actually look like, and what properties are lost when a 6D structure is projected onto a 2D page. Yau writes that he likes geometry in part because it is the most visualizable of mathematical disciplines. Yet when it comes to visualizing his most famous achievement, all we get is a brief description three chapters later.

Like science itself, science writing is an incremental process. Sometimes it takes a succession of books and articles before scientists and writers hit upon the clearest explanations. By bravely attempting to explain areas of mathematics that no-one has ever tried to relate to the public before, The Shape of Inner Space takes a huge step forward in this process. Even readers who never manage to finish the book will benefit indirectly, as it will undoubtedly influence how string theory is taught and written about in the future. Until then, however, Calabi–Yaus will still be little more than pretty pictures.