How do you study a phenomenon that cannot be replicated on Earth? You study one that has nothing to do with it, but looks incredibly similar mathematically. Matthew R Francis examines how these analogue systems are being used to study monopoles and black holes
Some experiments simply can’t be done. It’s a hard truth that physicists learn to face at an early stage in their careers. Some phenomena we want to study require conditions that are out of reach with our current techniques and technologies.
This is especially true when physicists make predictions about the very early universe. Theories hypothesize, for example, that certain particles may have been created during this high-energy period, but our colliders are just not powerful enough to replicate those conditions, which means we cannot create the particles ourselves. The physics that exists only in or around black holes poses a similar problem. Since these massive objects are very far away (the closest known is thousands of light-years distant) and would require hitherto unfeasible amounts of energy to make in the lab, we’re not able to test our theories about them.

At least, we can’t test them directly. Another way to look at these complex processes is by using “analogues”: experiments on systems that look radically different on the surface, but which reproduce the predictions of hard-to-test theories in some manner.
If the equations that describe a very complicated physical system are mathematically like those describing an experimentally accessible system (the analogue) then the outcomes of tests on the simpler system reveals something about the complex one and provides a necessary check on theories. However, analogues aren’t the same thing as a direct experiment, so we don’t always know if the mathematical similarity between two systems tells us if the theory we’re testing describes something real.
Mimicking monopoles
Richard Feynman, who is best known for his work in uniting quantum mechanics with electromagnetism, realized that many quantum systems are ideal analogues. For example, systems of interacting electrons in materials are often far too complicated to simulate on a normal computer, but physicists can create custom materials where the electrons and atoms interact in such a way to produce highly controllable quantum excitations known as “quasiparticles”. These quasiparticles can behave like everything from massless particles to electrons moving near the speed of light. Some of those might exist only as artefacts of the material, but others might correspond to theoretical predictions that are difficult to test with direct experiments.
Magnetic monopoles fall into that second category. Typically, magnetic fields are produced by moving or spinning electrically charged particles, and always come as dipoles: paired north and south poles. However, these hypothetical monopoles act like an isolated north or south pole of a magnet. In a famous thought experiment of 1931, Paul Dirac worked out the interaction between an electron and a hypothetical magnetic monopole particle. He discovered that the existence of a monopole – even a single one existing anywhere in the universe – could explain why electric charge is quantized, only occurring in multiples of the fundamental charge e carried by electrons (Proceedings of the Royal Society A 133 60).
Dirac’s theory was an exciting proof of principle, but it said nothing about how massive monopole particles should be. Later theories unifying the fundamental forces of nature predicted that monopoles should exist, but they also should have masses 1016 times that of the proton. Although “passive” detectors look for monopoles likely made in the early universe, even the most powerful particle colliders we have, or could build in the foreseeable future, can’t produce enough energy to make monopoles from scratch.
Mikko Möttönen of the University of Aalto in Finland and his colleagues realized, however, they could mimic monopoles using Bose–Einstein condensates, which are very cold systems of atoms that act together as a single entity described by a quantum wave function. By manipulating that wave function, the researchers could simulate several properties of monopoles – the first of which is how electrons behave in the presence of a monopole (Phys. Rev. X 7 021023).
“When we do the experiment on the [analogue] Dirac monopole, we find the wave function of a charged particle (it could be an electron) that is around the monopole,” says Möttönen. “That’s what we have got in the experiment: basically the properties of an electron interacting with the magnetic monopole. We have now produced a system that is an analogue system. It’s not the same system, but it has the same properties.”
While that experiment simulated the behaviour of a charged particle in the presence of a monopole, Möttönen and his collaborators performed a second set of experiments simulating the monopole itself. This took the form of a “topological defect” in the Bose–Einstein condensate: a sort of hole in the quantum wave function. The result of the monopole defect was a shift in the behaviour of the wave function around it, altering the quantum spin and phase of the field in the same way a magnetic field does. The properties of this “synthetic” analogue magnetic field were precisely what was predicted for a magnetic monopole (Nature 505 657).
“That was the first time ever experimentally people had observed a point-like topological defect in a quantum system,” Möttönen says. “That tells us on a general level that this kind of point defect does exist in nature.”
It was an important discovery, but as with the Dirac monopole experiment, Möttönen points out that the burden of proof still lies with the particle physicists. “It doesn’t guarantee that they exist as natural particles, as natural magnetic monopoles,” he says. “Do monopoles exist at all in quantum systems? This is certainly something we can say ‘yes!’ they do exist.”
To put it another way, magnetic monopole particles may or may not exist, but if they do, experiments by Möttönen and his colleagues show they likely behave the way theory predicts. It’s an important step and illustrates both the power and limitations of analogues.
Black hole in a bathtub
If magnetic monopole particles are out of experimental reach, black holes are even farther removed. We might get lucky if a monopole particle created shortly after the Big Bang drifts into our passive detectors, such as the IceCube observatory at the South Pole. But for obvious reasons we don’t want black holes to show up near Earth. Meanwhile, we’ll likely have to wait centuries for technology to be able to generate the energies needed to create a subatomic black hole in a particle collider.
However, we know black holes exist, while monopoles are still in the realms of theory. The problem is that there are many theoretical predictions about black holes that we can’t test directly. The most important of those phenomena are close to the event horizon: the boundary inside which nothing can escape the black hole’s gravity.
Most astrophysicists believe event horizons exist, but they have yet to be observed directly. Astronomers are processing data from the Event Horizon Telescope, which is a global network of telescopes that may provide the first clear image of the black hole at the centre of the Milky Way. However, even such an image won’t give us much particle-level information about the physics near the event horizon. Also, the Milky Way’s black hole is “supermassive”, which means gravity at its event horizon isn’t as intense as the gravity near a smaller black hole.
In a set of important papers in the 1960s and 1970s, Roger Penrose, Stephen Hawking, Jacob Bekenstein and others showed that certain physical processes can extract energy and angular momentum from black holes. What’s more, black holes interact with the quantum vacuum – the soup of virtual particles filling space–time – in a process that also extracts energy from the black hole, making them shrink. The end-point of that interaction, known as Hawking radiation, is the complete evaporation of a black hole.
However, Hawking’s prediction involved combining quantum calculations with general relativity, since there is no quantum theory of gravity he could use. In other words, it’s an approximation to an unknown underlying theory governing black-hole behaviour, and it may or may not describe reality. And without a laboratory black hole, we can’t test the ideas directly.
The equations governing small ripples on flowing water are very close to those for particles moving along trajectories governed by gravity, as described by general relativity
Then in 1981 William Unruh realized ordinary water could provide an analogue for black-hole interactions and evaporation (Phys. Rev. Lett. 46 1351). He found the equations governing small ripples on flowing water were very close to those for particles moving along trajectories governed by gravity, as described by general relativity. Even more strikingly, the analogue system produced behaviour akin to Hawking radiation and other processes by which quantum particles interact with a black hole. And physicists understand fluid dynamics of this type very well.
It’s an astounding analogy, since water resembles a black hole even less than a Bose–Einstein condensate resembles a magnetic monopole. But it’s similar, in that the equations governing the particles are mathematically similar, even if the physical systems are radically different.
Silke Weinfurtner of the Quantum Gravity Laboratory at the University of Nottingham in the UK is one physicist using such analogues to look at black holes. “I have a set of equations, [and] I have a physical process I want to describe,” she says. “In the analogues, we find the same set of equations [describing a different system], approximately.”
But, Weinfurtner adds, she is more interested in the effects than mimicking exactly a particular space–time geometry. “We want to have something that has the same [event] horizon structure.”
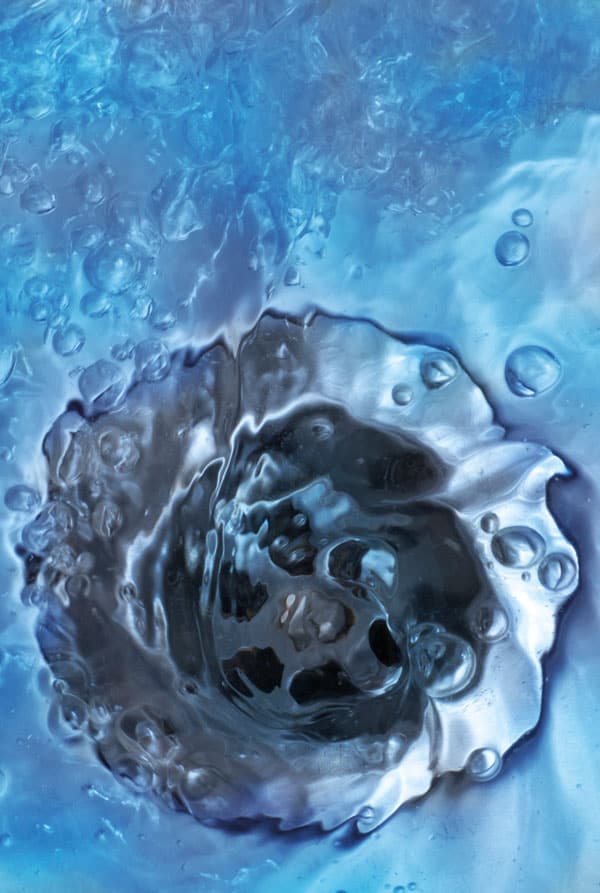
The experimental realization of Unruh’s idea involves a narrow channel, with water flowing in at a controlled rate. The water drains out through a plughole, much like a bathtub, and the rotating vortex simulates the effect of black-hole rotation. This “background” flow is the analogue to the space–time geometry of gravity. A wave generator creates ripples on top of this background flow, which represents particles moving under the influence of gravity.
“Closer to the vortex flow, there is an area where the fluid flow is faster than the ripples can propagate,” says Weinfurtner. “That’s where you have your analogue event horizon. If a little ripple crosses the effective event horizon, it has no choice but to get drained.”
Weinfurtner and her colleagues simulated particles scattering off the analogue event horizon following Unruh’s general scheme, and observed several phenomena analogous to those predicted near black holes. One of these, published this year, was a simulation of Hawking radiation (Phys. Rev. D 97 025005), where the ripple splits into two pieces with positive and negative energy; the positive-energy ripple escapes, while the negative-energy ripple crosses the event horizon, reducing the total energy of the flow inside the “black hole”. Other experiments modified the vortex, analogous to how angular momentum can be extracted from black holes via the Penrose process (Nature Phys. 13 833).
It’s a beautiful result, but the fluid-dynamics equations governing the system approximate the Hawking theory – which itself may not correspond to physical reality, since it doesn’t come from a complete quantum theory of gravity. “We do not know if that framework truthfully applies to black holes,” Weinfurtner admits. “It’s an approximation of the astrophysical black hole. It’s an ad hoc equation. We didn’t find the analogy that this is a black hole, it’s more like field fluctuations around a black hole.”
In other words, it’s an approximation to an approximation. How well it holds up has yet to be determined.
Not just a metaphor
Weinfurtner’s research examines analogues of systems where even our theories are incomplete. “We think black holes can lose mass through Hawking radiation, but we have not verified that it’s true,” she says. “We have a set of equations, and that gives that prediction. But we have not verified that effect, which means we also haven’t really verified the whole framework. We don’t know if that set of equations actually gives us the right physics.”
These experiments aren’t equivalent to studying “the real thing”, but that’s not to say they don’t tell us anything at all
For the magnetic monopole, Hawking radiation and other analogue experiments, it’s easy to go too far either way in interpretation. On the one hand, these experiments aren’t equivalent to studying “the real thing”, but that’s not to say they don’t tell us anything at all. The results are enough to say if these theories correspond to reality, the analogues show how they work.