Two independent teams of physicists in the US have entangled three superconducting quantum bits (qubits) for the first time. Entangled trios are of particular interest to those building quantum computers because three is the minimum number needed to do quantum error correction – which is needed to keep quantum computers running.
Despite the promise of outperforming conventional computers on certain tasks, quantum computers can only be useful if physicists can work out how to entangle a relatively large number of qubits. So far researchers have managed to entangle as many as eight ion qubits and 10 photon qubits. But when it comes to entangling superconducting qubits, the limit so far has been just two.
Although they are difficult to entangle, superconducting qubits could have several advantages. In particular, they are completely solid state, which means that they are robust and can be implemented much like conventional electronic devices.
The new trios of entangled superconducting qubits were created by John Martinis and colleagues at the University of California, Santa Barbara, and by Robert Schoelkopf and his team at Yale University. The Santa Barbara team had previously devised a way of entangling two superconducting qubits back in 2006, while last year the Yale researchers had executed several quantum-computing algorithms using two entangled superconducting qubits.
Entangled transmons
The Yale physicists used qubits called “transmons”. Each transmon is made from two tiny pieces of superconductor connected by two tunnel junctions. The superconductors contain a large numbers of “Cooper pairs” of electrons that can move through the material without any electrical resistance.
The energy levels of the qubit are defined by the precise distribution of Cooper pairs between the two pieces of superconductor. One such energy state is denoted a logical “0” and another state “1”. Transitions between these two states occur by absorbing or emitting a microwave photon.
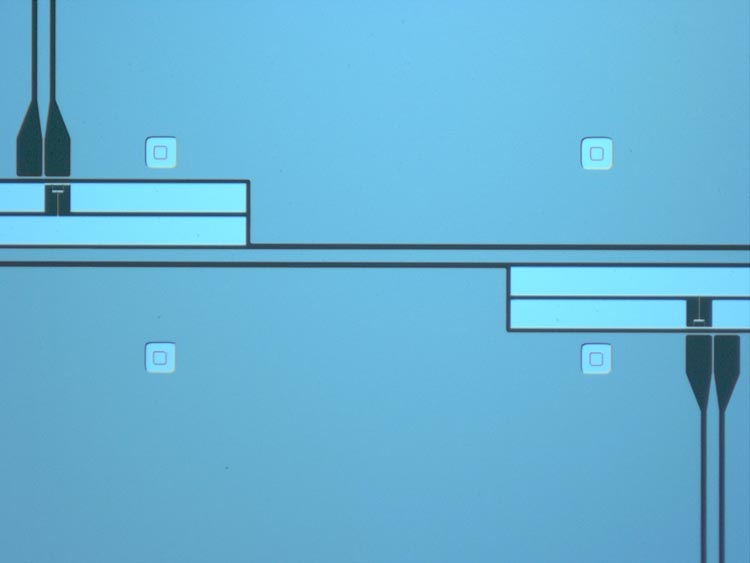
The Yale team made a chip containing four transmons that are coupled to a microwave waveguide. The four transmons are arranged as two two-qubit controlled-phase (C-phase) logic gates.
Making a GHZ state
The gates are used to entangle three of the qubits and create a Greenberger–Horne–Zeilinger (GHZ) state. This is a superposition of the state in which all three qubits are “0” and the state in which all three qubits are “1”. The GHZ state is made by first entangling two qubits using one C-phase gate. The other gate is then used to entangle the third qubit with the entangled pair.
The team verified the entanglement using quantum-state tomography. This involves creating the GHZ state, measuring the values of the qubits – and then repeating the entire process many times over. This is necessary because an individual measurement of the qubits puts the system into one of many possible states. Multiple measurements are needed to map out the probability that the system is in a certain state.
Oscillating phase
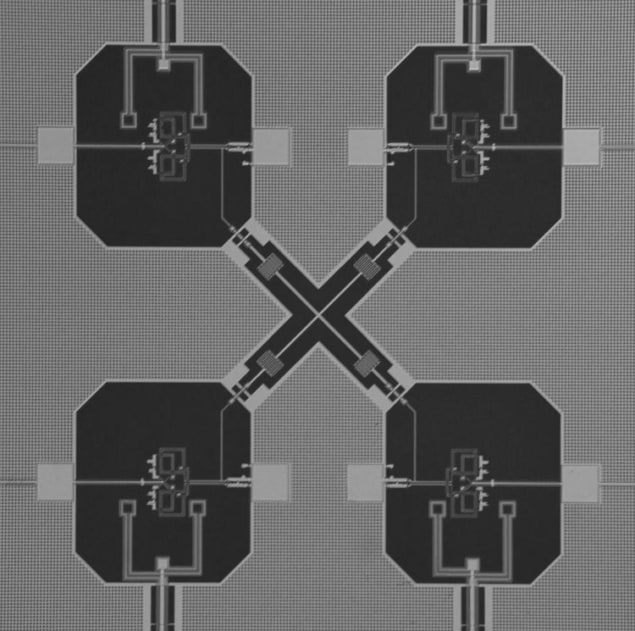
Meanwhile in Santa Barbara, Martinis, Matthew Neeley and colleagues used superconducting phase qubits to achieve three-qubit entanglement. A phase qubit is a single Josephson junction, which comprises two pieces of superconducting metal separated by a very thin insulating barrier. The two logic levels are defined by quantum oscillations of the phase difference between the electrodes of the junction.
The team created its GHZ state using a similar technique to the Yale group – except that it configured the microwave circuit to form two controlled NOT (CNOT) gates rather than C-phase gates.
The Santa Barbara group then reconfigured its circuit to create another type of entangled state called the “W” state. This is a superposition of the three states in which one of the three qubits is “1” and the other two qubits are “0”. “To create this state we excite just one qubit, putting a single quantum of energy into the system,” explains Neeley. “We then turn on a coupling interaction between all the pairs of qubits, which spreads that one excitation out among the three qubits.”
This interaction is created by connecting the qubits together using a network of capacitors. If the interaction is left on for just the right amount of time there is an equal probability of finding the excitation in any of the three qubits, Neeley told physicsworld.com.
Useful in different ways
Both the GHZ and W states are expected to play important roles in quantum computing. According to Neeley, W states are relatively robust compared to other entangled states. This is because the destruction of the quantum nature of one qubit does not necessarily destroy the entanglement of the other two qubits
GHZ could be particularly useful for quantum error correction, which protects a quantum computation from the destructive effects of noise. If one of the three qubits is inadvertently flipped, for example, this can be corrected by determining the value of the other two qubits.
“Error correction is one of the holy grails in quantum computing today,” explains Robert Schoelkopf. “It takes at least three qubits to be able to start doing it, so this is an exciting step.”