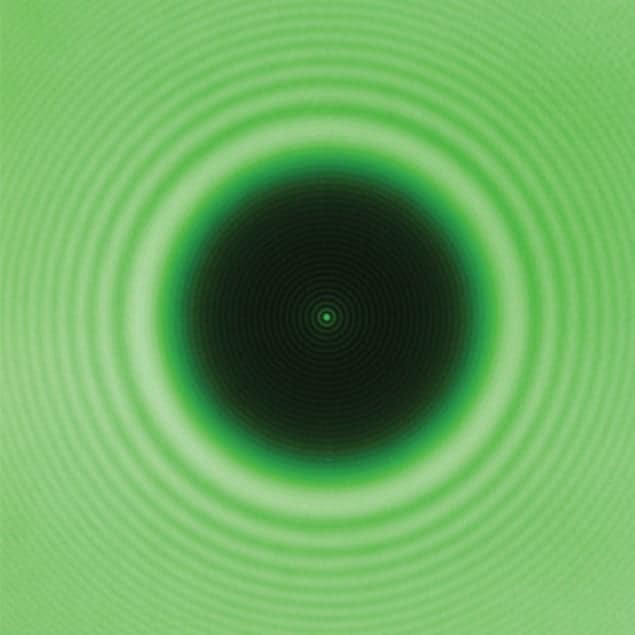
“It’s an extraordinarily simple set-up,” says Hal Metcalf as he switches on a small laser pointer, creating a bright green spot about 2 mm in diameter on a wall 10 m away. He then clamps an upright nail to a stand and puts a magnet on its head, before carefully suspending a ball bearing from its tip.

Placing this stand between the laser and the wall, Metcalf adjusts the beam to hit the ball bearing. Its shadow is now surrounded by a glowing green halo, the whole resembling a miniature solar eclipse. Except for one thing: in the dead centre of the shadow is a green spot, glowing as brightly as if the ball were not there. Unbelievable!

A pioneer of laser cooling and a distinguished teaching professor at Stony Brook University, Metcalf gives this demonstration in his third-year optics course and in the university’s Laser Teaching Center (LTC). He loves it. “There’s no tricks. No lenses, no mirrors, nothing! Just a laser beam and small ball bearing.”

It is one of several demonstrations that he and LTC director John Noé use to motivate students. Metcalf and Noé do them without using mathematics, until the students get curious enough about the mysterious happening to ask how to describe it. The ensuing conversations usually go like this:

Metcalf: “It’s easiest not to use English for the description but mathematics.” Student: “Really? What do I need?” Metcalf: “Well, differential equations or matrix algebra or calculus…” Student: “That’s all?”

“You have to motivate students to want the maths,” Metcalf adds. “I hate it when I hear, ‘This happens because it must satisfy that equation.’ No it doesn’t. This happens; that equation describes it.”

But this demonstration – I’m not going to name it, for reasons I’ll discuss – is not just a dramatic teaching aid. It is also an instructive episode in the ambiguities of discovery.

Fresnel, Poisson, Arago

At the start of the 19th century, the prevailing theory of light was Newton’s “corpuscular” or particle theory, according to which light travels in straight lines. But in about 1807 the British scientist Thomas Young demonstrated the wave-like nature of light through its interference effects. Opinion over the true nature of light was divided, not least among members of the French Academy of Sciences, most of whose members championed particles.

At the time, the academy held periodic competitions in a kind of miniature “grand challenge” series whose aim was to clarify controversial matters. And in 1818 it declared that diffraction would be the topic of the competition for the following year.

Enter Augustin-Jean Fresnel. In 1818 Fresnel was supporting himself as an engineer in Rennes, but seized every opportunity to travel to Paris to craft a wave theory of light inspired by Young’s observations. He developed a way to calculate the net effect of a set of waves by resolving each wave into two components, adding the components and combining the results. Barely making the deadline, Fresnel entered the academy’s competition with an essay proposing a theory that, he claimed, could allow the light intensity at any point behind a diffracting object to be calculated.

The committee charged with judging the competition was, however, dominated by partisans of the particle theory, including Siméon-Denis Poisson, who were sure Fresnel’s theory was flawed. In fact, Poisson noticed what seemed to be a show-stopper. According to Fresnel’s work, if light were shone on a circular obstruction, a bright spot would appear in the centre of the shadow, as bright as if the obstruction were not there at all. Obvious nonsense! Not only that, Fresnel’s equations indicated that light shining through a circular hole could produce a dark spot in the middle.

The committee’s head was, however, François Arago – one of the few French scientists besides Fresnel acquainted with Young’s work, and therefore able to appreciate Fresnel’s contributions. Arago carried out the experiment with a flame, filters and a 2 mm metal disc attached to a glass plate with wax. To everyone’s surprise, and Poisson’s chagrin, Arago observed the spot and Fresnel won the competition.

The episode was deeply satisfying to Fresnel, even if he had little patience with mere praise. “All the compliments that I have received from [committee members] Arago, [Pierre] Laplace and [Jean-Baptiste] Biot,” he wrote to Young a few years later, “never gave me so much pleasure as the discovery of a theoretic truth, or the confirmation of a calculation by experiment.”

If the demonstration is so simple, I asked Metcalf, why wasn’t it discovered earlier in things like eclipses? “The Moon’s not nearly round enough,” he snorted. “All those mountains! The Sun’s not a point source of coherent light. People didn’t always have laser pointers.”

The critical point

The episode illustrates the ambiguities of discovery. Who’s the discoverer? Fresnel, who produced the original framework? Poisson, who showed the spot was a direct consequence but was firmly convinced that it didn’t exist? Arago, who did the experiment? Moreover, two other scientists turned out to have noticed the spot a century earlier but did not know what to make of it.

What about the French Academy, whose actions set the discovery in motion? Didn’t Young play a role? Even Newton? Moreover, the spot is just an illustration of a more general phenomenon that complementary obstruction patterns produce complementary diffraction results, described by Jacques Babinet’s theorem.

To pick out any one person, or combination, may be useful in education, in naming the phenomenon, or in dishing out awards. But philosophically, it’s sloppy – like designating the most valuable player in a sports competition as the person who won it. The rigorous answer to the question “Who discovered that spot?” is the entire scientific community.