The chaotic nature of weather could be turned to humanity’s advantage by allowing a series of tiny changes to keep weather systems on track. That is the vision of two scientists in Japan who have used computer simulations based on a butterfly attractor to show how even the smallest perturbations could prevent extreme events such as tornadoes or heavy downpours – assuming they are able to confirm their idea with more realistic modelling.
Scientists have been experimenting with weather manipulation for decades and there have been numerous attempts to induce rainfall by releasing aerosols into the atmosphere from aircraft or ground stations to stimulate condensation of water vapour. But while advancing our knowledge of cloud physics, such trials have yielded mixed results when it comes to their practical exploitation, according to Takemasa Miyoshi and Qiwen Sun at the RIKEN Center for Computational Science in Kobe.
The two researchers point out that there are also major efforts under way to investigate the feasibility of altering the climate via geoengineering. But these proposals – including the launching of giant mirrors into space or the dispersal of dust in the upper atmosphere – are controversial given their potential for unintended side effects.
Chaotic control
Miyoshi and Sun have taken a different approach. Rather than attempting to induce irreversible changes to nature, they say that they aim to “control the weather within its natural variability and to aid human activities” – such as by shifting a specific weather system spatially so that rain can be dumped where it does less damage. Their tool in this endeavour is chaos – the fact that chaotic systems are extremely sensitive to changing inputs. “If the proper infinitesimal perturbations are within our engineering capability, we could apply the control in the real world,” they say.
Their work makes use of the simplified system known as Lorenz’s butterfly attractor. Edward Lorenz was an American mathematician and meteorologist whose work on chaos theory inspired the popular idea of a butterfly flapping its wings in Brazil and setting off a tornado in Texas. The butterfly in this case illustrates the same basic idea of a chaotic system’s sensitivity to initial conditions, although the name refers to the shape of a region bounding possible trajectories in phase space.
Lorenz formulated a set of three differential equations to describe in simple terms the convective properties of a layer of fluid in the atmosphere. The equations feature three variables – the rate of convection as well as the horizontal and vertical temperature variation – which are represented as the positions along three orthogonal axes. The equations describe a chaotic system that evolves by tracing out a series of orbits that resemble a butterfly – having two wings connected in the middle. Although the system remains confined to that region of phase space, it can suddenly and unpredictably flip from one wing to the other.
Side-by-side simulations
The aim of Miyoshi and Sun was to establish whether infinitesimal perturbations to a chaotic Lorenz system could keep it confined to just one of the butterfly wings – and thereby avoid dramatic changes in the weather. They did so by running two simulations side-by-side, the first representing unmodified nature while the second, starting from the same initial conditions, they controlled using imperfect knowledge of the former to try and avoid sudden shifts to the other wing.
The researchers ran their simulations using discrete time steps. At every eighth step they updated the second system with noisy information about the natural system’s current coordinates in phase space, then used an ensemble of three models to provide a revised estimate of where the system would end up at a given point in the future. Were any of the models to predict that the system would switch wings, they would then change the coordinates of the controlled system by the same tiny amount at each step until next observing the current state of “nature”.
Each computational experiment carried out by the researchers involved 1000 such cycles, with 40 experiments being run for each of a range of model time horizons and perturbation sizes. By modelling far enough out to the future, they found they could keep the controlled system in the same wing at least 80% of the time, despite nature flipping wings. The perturbations, meanwhile, needed to be not too big and not too small to achieve the best results.
Cost and energy expenditure
Having shown that their chaos-based approach can in principle be used to control the weather, Miyoshi and Sun now plan to apply the technique to more realistic weather simulations. They also say they need to investigate just how small interventions could be in terms of cost and energy expenditure to make a difference to extreme weather events.
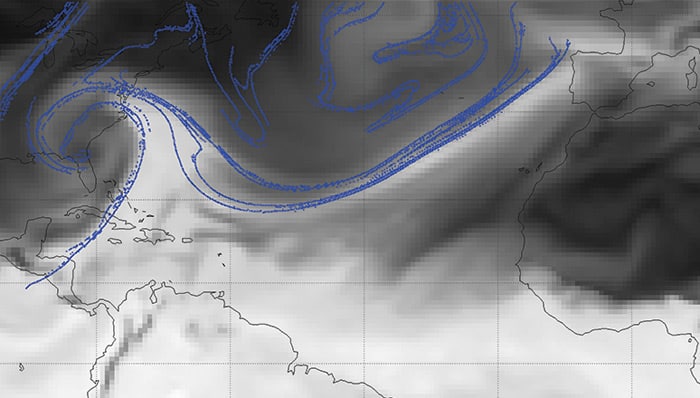
New study could help predict floods caused by atmospheric rivers
Above all, they say that caution is needed. They point out that keeping nature on one wing of the Lorenz attractor may not necessarily guarantee success – it being possible that extreme events could occur without switching sides. “We must consider and assess every potential impact caused by the control and have proper protocols for social, ethical, and legal agreement about real-world operations,” they say.
Eugenia Kalnay at the University of Maryland in the US suggests that perturbations could in principle be generated by increasing or decreasing the drag created by wind turbines in specific ways over short periods of time. “One could imagine reducing the precipitation during a heavy flooding event, or increasing the precipitation during a drought,” she says, stressing that this example is hypothetical.
The research is reported in Nonlinear Processes in Geophysics.