Henrik Melbéus and Tommy Ohlsson describe three different theories of extra dimensions – universal, large and warped – and how these unseen dimensions could be observed, if they exist at all

We experience three spatial dimensions in nature: length, width and height. In addition, we perceive time as a fourth dimension. But some theoretical physicists have speculated that “extra” spatial dimensions could exist in addition to the four ordinary space–time dimensions, although they are too small to see with the naked eye. Now, with the advent of new data from the Large Hadron Collider (LHC) at CERN near Geneva in Switzerland, as well as other particle- and astroparticle-physics experiments, it might be possible to answer the fundamental question of whether these extra spatial dimensions exist.
The idea that space–time could have more than four dimensions was first proposed by the German mathematician and physicist Theodor Kaluza and the Swedish theoretical physicist Oskar Klein in the early 20th century. In 1921 Kaluza published an article in which he extended Einstein’s theory of general relativity (which is still the best known description for gravitation) from four to five dimensions, and in 1926 Klein assumed that an additional fourth spatial dimension is curled up into a circle with an extremely small radius – the extra dimension bends round on itself and is said to be “compact”. Indeed, it can be shown that 5D space–time can be separated into Einstein’s theory of gravitation in four dimensions and Maxwell’s theory of electromagnetism. For this reason, a physical model that tries to unify the fundamental forces of gravitation and electromagnetism is known as a Kaluza–Klein theory (KK theory), although nowadays the phrase is used to refer to any theory with extra spatial dimensions.
Trying to imagine extra spatial dimensions is not at all intuitive. How could there be anything other than front-to-back, side-to-side and up-and-down. One analogy to help understand this is to consider a tightrope (figure 1). Seen from a distance, such as from the eyes of a human being balancing on the rope, it might seem possible to move only forwards or backwards. However, on closer inspection, such as from the perspective of an ant, it is possible to move along the rope and also around it. In the same way, extra dimensions could be hidden for someone who is looking at them from a large distance compared with their size.
As for how extra dimensions could reveal themselves, the experimental study of fundamental particles is the most promising route. The energy of a particle in a 3D space consists of the rest energy of its mass, E = mc2, and the kinetic energy of its motion. If extra dimensions exist, the particle will have more freedom to move and so could obtain an additional, independent contribution to its kinetic energy. Since we do not observe the motion of the particle along the extra dimension, we would interpret this kinetic energy as part of its rest energy, or in other words the mass of the particle. To us, the particle would not look like one particle, but a set of particles – all with different masses. The faster the particle moves along the extra dimension, the larger this apparent mass seems to be. It turns out that the mass of each particle is related to the mass of the particle at rest in the extra dimension. We assume that the Standard Model particles are at rest in the extra dimension, and that for each of these known particles there could exist heavier versions that have not yet been discovered. Known as “KK particles”, we can arrange each set of them in a schematic “KK tower” with KK (particle) numbers n = 1, 2, 3… and masses mn = √(m2 + n2/R2), where m is the mass of the Standard Model particle (n = 0) and R is the characteristic size of the extra dimension. For example, one can imagine an electron, which is a Standard Model particle, and a corresponding KK tower of heavier KK electrons.
KK theories are interesting for several reasons. Perhaps most importantly, they can be used to address several of the shortcomings of the Standard Model of particle physics, which is currently our best description of the subatomic world. Although the Standard Model is very successful, it does have a number of problems that point towards the need for it to be extended. KK theories are one possible such extension; others include, for example, supersymmetry, which predicts that for each of the Standard Model particles there exists a heavier “sparticle” sibling, and grand unified theories, in which the strong, weak and electromagnetic interactions are regarded as different aspects of a single force.
Standard Model shortcomings
Among the most famous problems with the Standard Model is that observations of how galaxies move give us a large amount of evidence for there being more matter in the universe than we can see. This additional matter has been termed “dark matter”, although “invisible matter” would perhaps be a better term, since it is not visible (not “luminous”) as ordinary matter is.
The current most plausible solution to this problem is that dark matter is made up of particles that interact very weakly with light. From the particle physics point of view, however the problem with this solution is that none of the known particles in the Standard Model could make up the dark matter. But in some KK theories, it turns out that some of the KK particles that are predicted to exist could be this elusive dark matter as they would not interact with light and would have other characteristics that we expect of dark matter.
Another peculiarity in particle physics is the fact that gravity is much weaker than all of the other fundamental forces. This force is nevertheless important because, unlike the strong and weak forces, it has an infinite range, and most macroscopic objects are electrically neutral and so are not strongly affected by the electromagnetic force, leaving gravity as the only important force for them. From the point of view of quantum physics, the weakness of gravity is a puzzling fact that would seem to require a very precise fine-tuning of the parameters in nature. But in KK theories, the weakness of gravity might be fundamental.
A third problem relates to the properties of neutrinos in the Standard Model. Being electrically neutral and interacting very weakly with other matter, neutrinos are very difficult to detect. In fact, the Standard Model says that all neutrinos are massless. However, observations of neutrinos turning from one form into another – a phenomenon known as “neutrino oscillation” – strongly indicate that neutrinos do have mass. We would therefore have to extend the Standard Model to take this fact into account, but the problem is that neutrinos are much lighter than all other known (Standard Model) particles. In KK theories, natural mechanisms that generate these small neutrino masses can be obtained.
Universal pictures
The bottom line is that to have avoided detection thus far, any extra dimensions would have to be compact and small. Indeed, one might naively imagine that extra dimensions must be so small that we will never be able to observe them, at least in the foreseeable future. However, in recent years different models have been proposed that avoid such stringent constraints, allowing models of extra dimensions to be tested in high-energy physics experiments.
There are three main models that describe extra spatial dimensions. In the scenario of universal extra dimensions (UED model), which was proposed in 2001 by the theoretical physicists Thomas Appelquist, Hsin-Chia Cheng and Bogdan Dobrescu (Phys. Rev. D 64 035002), all of the particles of the Standard Model are allowed to propagate in the extra dimensions, with each fundamental particle having its own KK tower. In this model, there is an extra number (comparable to a particle’s mass or charge) attached to each particle known as “KK parity”, which is assumed to be conserved in particle reactions, meaning that the product of all particles’ KK parities before a reaction should be equal to the product of all remaining particles’ KK parities after the reaction. The KK parity +1 is assigned to all even-numbered KK particles, which includes all Standard Model particles as they have a KK number of 0, whereas odd-numbered KK particles have a KK parity of –1. The consequence of conservation of KK parity is that odd-numbered KK particles can only be produced in pairs, which has a low probability of happening despite being the only allowed option. Furthermore, a second-order KK particle (with parity +1) can decay into two Standard Model particles – because (+1) = (+1)(+1) – but a first-order KK particle (with parity –1) cannot decay into any number of Standard Model particles because –1 never equals (+1)n.
The UED model would give rise to observable effects at the LHC such as the production of two Standard Model particles or more complicated signals, as would the ADD model described below. Another important implication of KK parity is that it ensures that the so-called lightest KK particle (LKP) is stable and hence could be a possible dark-matter candidate. This kind of dark matter is known as Kaluza–Klein dark matter.
Going extra large
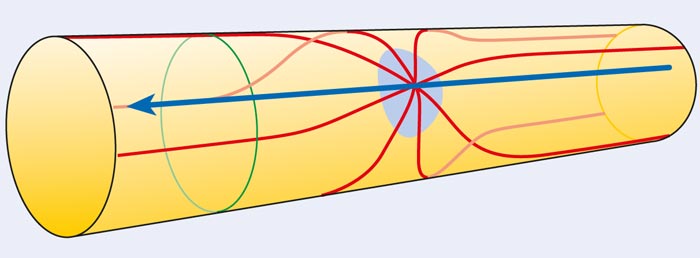
The second significant model describes “large extra dimensions”, which were first proposed in 1998 by Nima Arkani-Hamed, Savas Dimopoulos and Gia Dvali, in what is known as the ADD model (Phys. Lett. B 429 263). The novel feature of this model is the assumption that the Standard Model particles are confined to a so-called brane, which is identified with ordinary 4D space–time, but residing in a larger space–time (figure 2). An analogy is the surface of the Earth being a 2D layer that resides in a larger 3D world. In this case, the surface of the Earth would be the brane.
Since Standard Model particles only “live” in the usual dimensions, not in the extra dimensions, they will not help us in setting limits on the size of extra dimensions. On the other hand, the particle responsible for gravity, the graviton, is not a Standard Model particle and is therefore allowed to propagate in the extra dimensions. In principle, the assumption that gravity lives in a larger space–time leads to deviations from Newton’s gravitational law at short distances. However, because of its weakness relative to the other fundamental forces, gravity has only been tested down to distances of the order of microns, and thus the experimental constraints are still quite weak.
Warped thinking
In addition to the ADD model, the theoretical physicists Lisa Randall and Raman Sundrum proposed a similar model in 1999 (Phys. Rev. Lett. 83 4690). This is normally called the Randall–Sundrum (RS) model but is sometimes called the 5D warped geometry theory, and it assumes that the real world is a higher-dimensional universe described by a warped geometry instead of a flat geometry as in the case of the ADD model. (A warped geometry is one in which space is curved, as in Einstein’s general relativity.)
In the ADD and RS models, the weakness of gravity compared with the other fundamental forces is related to the geometry of space–time. Simply speaking, gravity is spread out in a larger space–time than the other forces, which act only in the 4D brane. In fact, this means that all of the four forces could have similar strengths and gravity only appears weaker as a result of this geometric dilution.
One final framework that implies extra dimensions is string theory, though this is very different from and more hypothetical than typical KK theories. This mathematical approach attempts to describe particles as vibrations of tiny strings and predicts that the number of space–time dimensions has to be 10 or 11, depending on the exact formulation of the theory.
The hunt is on
The search for extra dimensions is not just something for the future but is currently ongoing at the LHC. If extra dimensions exist, one could hope to produce KK particles by colliding ordinary particles (protons in the case of the LHC) at very high energies. So far neither ATLAS nor CMS – the LHC’s two main experiments – has found any signs of extra dimensions. However, these non-observations are still useful. The fact that nothing has been observed places stronger constraints on the size of the extra dimensions: if they exist and have not yet been seen, they must simply be even smaller than was previously thought to be necessary.
So far, new constraints have been placed on the ADD and RS models by the ATLAS and CMS experiments. For the ADD model, they have found that the so-called effective extra-dimensional Planck scale, which is the highest possible energy scale that makes physical sense within the given model, must be larger than 2–4 TeV, whereas for the RS model, the graviton needs to be heavier than 1–2 TeV. As the energy scale of a KK particle, i.e. its mass, is essentially directly proportional to the inverse size of the extra dimension, this equates to finding a maximum length scale for the extra dimension.
On 4 July this year the ATLAS and CMS collaborations at CERN announced that they had discovered a new particle with a mass of around 125 GeV, which most likely is the famous Higgs particle. If it is the Higgs, then the characteristic size of the extra dimension needs to be smaller than about 1.8 × 10–18 m – about a thousandth of the proton radius. But it should be noted that the LHC has really only just started collecting data, and much could remain to be discovered. Previously, other, less powerful machines have been used to restrict extra dimensions in a similar way, most notably the former accelerators the Large Electron–Positron collider at CERN and the Tevatron at Fermilab in the US.
Limits on extra dimensions could also be identified by the many dark-matter experiments, such as the XENON detector at the Gran Sasso underground laboratory in Italy, the space-borne Fermi and PAMELA satellites, and the IceCube neutrino telescope at the South Pole. Indeed, this year the Fermi collaboration put strong limits on the Planck scale in the ADD model that are compatible with the LHC results: it has to be larger than 230 TeV for two extra dimensions, 16 TeV for three and 2.5 TeV for four. If dark matter is made up of KK particles – i.e. Kaluza–Klein dark matter – then these experiments could prove useful in the study of extra dimensions.
Although neither the LHC nor any dark-matter experiments has yet found conclusive signs of physics beyond the Standard Model, over the next few years the LHC will produce numerous new data that will hopefully show whether extra dimensions exist or not. We may know the answer before the end of this year. Even if we do not, other experiments will continue the hunt, during what is proving to be a very interesting time for physics research at its most fundamental.