Andreas Schadschneider knows a thing or two when it comes to evacuating stadiums. Here he explains how models of pedestrian dynamics borrow ideas from physics to model people as particles

As 50,000 dejected England supporters watch their team trudge off the pitch after crashing out of the FIFA World Cup, fans console each other with pats on the back and talk of future glory, while comforting children with tear-stained red-and-white faces. But their thoughts soon turn to a new issue: how to get out of the stadium. As the venue’s architects will have designed exit routes for just this purpose, leaving is not usually a problem. But if fans need to evacuate fast because of, say, a bomb threat or other emergency, even the best exit strategies can become obsolete. If only two exits are available out of four, can the streams of spectators be redirected in such a way that nobody gets hurt? Worryingly, we cannot definitively say “yes”.
However, a new project called Hermes, funded by the German Federal Ministry of Education and Research, aims to protect and save lives by developing an “evacuation assistant” that could allow stadiums and other venues hosting large events to be cleared quickly and more safely than is possible now. The Hermes system is designed to use information about a current situation to predict what will happen when extrapolated to the future. It involves feeding data into a computer program about the availability of rescue routes and the distribution of people as determined using video cameras linked to image-processing software. Computer algorithms modelling crowd dynamics are then used to predict potentially dangerous situations such as bottlenecks, allowing evasive action to be taken to stop these from happening.
But why are physicists like me involved in this research? The answer is that modelling pedestrians has a lot in common with modelling some other physical systems (see “Particle people” box). In particular, a crowd can be treated like a many-particle system, and ideas from statistical physics can be used to model collective effects without having to know the physiological and psychological features of each individual. Pedestrian dynamics is, however, a complex subject, although the phenomena behind how a crowd moves are simple enough to understand. After all, we are all pedestrians ourselves, moving among other people on a daily basis and making countless split-second decisions about where to go next.
Pedestrian traffic jams
The simplest (and most annoying) example of collective behaviour is when people get clogged up at bottlenecks. Pedestrian jams like these are the consequence of a simple exclusion principle: no two people can occupy the same space at the same time. Bottlenecks are simply areas where the capacity to accommodate people is reduced locally, such as exits or narrowing corridors. But they can also occur in less obvious, dynamic structures, such as when two or more streams of pedestrians merge. Identifying bottlenecks is an important task for safety analysis because such jams limit the maximum possible flow of people and thus strongly influence evacuation times.
One self-organization phenomenon that can frequently be observed in everyday life (watch out next time you go shopping!) is the dynamic formation of lanes. These occur in “counter flow”, where two groups of people move in opposite directions.
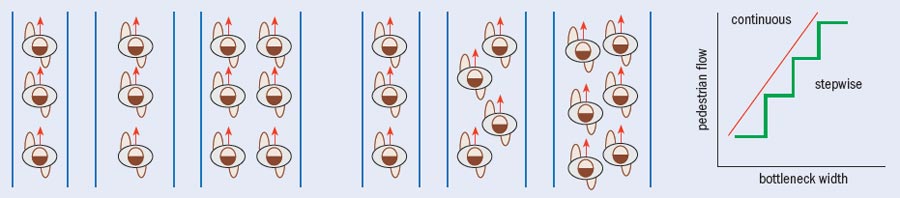
By self-organizing into lanes, walkers avoid interactions with oncoming pedestrians and can thus move faster than is otherwise possible. This happens effortlessly and requires neither communication nor a preference for moving on either the left or right. However, such a preference does exist empirically, with the favoured side usually corresponding to the side of the road people drive on. Another observation, which is somewhat surprising, is that the total flow in a counter-flow situation can be larger than if one of the lanes is turned around and everybody moves in the same direction.
When flows intersect at an angle other than head on, patterns of diagonal lane stripes can form in which clusters of pedestrians move in the same direction and with the same speed. Another intersecting flow phenomenon is the formation of short-lived “people roundabouts” that make the motion more efficient. Even if these require pedestrians to detour around the roundabout rather than walking in a straight line to their destination, they allow a “smoother” motion and an overall shorter journey time.
When counter flow and bottlenecks are combined, such as at crowded doors, the direction of motion is oscillatory. In other words, once one pedestrian is able to pass the bottleneck it becomes easier for others to follow in the same direction, until somebody is able somehow to squeeze through in the opposite direction and the flow direction changes again.
Being in an extremely dense crowd can be very unpleasant. The forces can quickly change direction and push you around like a helpless punchball without you being able to do anything about it. Such situations can lead to tragedies as people who stumble and fall are often not able to get back onto their feet. A famous example of this is the “hajj” pilgrimages to Mecca, which have seen more than a thousand pilgrims die over the last 20 years.
Putting people under the microscope
When it comes to designing exit routes from stadiums, it is important to know how the pedestrian flow at bottlenecks changes as the constriction gets wider or narrower. Although studies of this flow–width dependence go back to the beginning of the last century, even qualitative models are still controversial. Some experiments have been interpreted as flow increasing in a stepwise fashion with width, where flow only increases if an additional lane is formed. However, other experiments suggest a continuous increase of flow with width, which can be understood by the “zipper effect”.
To find out what is really going on, several lab experiments have recently been performed to study pedestrian bottlenecks, crossing streams and an important characteristic for building design called the “flow–density relation”. The latter is the number of people passing a fixed point during a certain time interval (the pedestrian flow rate) as a function of pedestrian density. In such experiments, researchers would ideally like to know the trajectories of every participant, from which, in principle, most quantities of interest could then be derived. However, in experiments with 250 individuals it is rather difficult to extract people’s trajectories by simply watching video footage. Therefore, researchers led by Maik Boltes at the Jülich Supercomputing Centre in Germany have developed special software called PeTrack (pedestrian tracking) that allows the trajectories of individuals to be determined automatically. The software currently requires the test subjects to wear a particular colour on their head for detection purposes, but future versions might enable tracking without such markers.

To find out what happens in a real sports stadium, the Hermes research group recently performed experiments in the Esprit Arena – home to the German second-division football team Fortuna Düsseldorf – using more than 350 volunteers, most of whom were students. We wanted to understand how the stands empty, how large groups of people behave on stairs and how different flows merge and interact. The results helped us to identify interactions between pedestrians and also how people respond to their physical surroundings. This information is essential for developing an accurate, calibrated model that can be used for safety analysis.
While experiments under non-evacuation conditions are vital to our understanding of pedestrian dynamics, we do also need to know what happens in an emergency, as there may be major differences. The problem is that empirical studies of emergency situations are very hard – even under lab conditions. Indeed, if they are made too realistic, they can be dangerous. Nevertheless, such experiments are sometimes necessary. New aeroplane designs, for example, have to pass an evacuation test in which all passengers can get off the plane in less than 90 s. In the certification trials for the Airbus 380, more than 30 of the almost 900 participants were injured; fortunately, only one injury was serious (a broken leg).
Naively, one might assume that passengers will be able to leave a plane faster if they are highly motivated. However, this is not always the case and the evacuation time depends strongly on the width of the exit. For narrow exits, it turns out that when passengers co-operate, they can exit faster than when they compete with one another to get off. But for wider exits, the opposite is true.
The reason for this surprising observation is friction – not just physical contact (people getting stuck), but also psychological effects such as moments of hesitation where different pedestrians want to move to the same destination but are not sure who should go first. Since no two people can occupy the same space simultaneously, these conflicts have to be resolved in some way. Indeed, modelling dynamics, especially near bottlenecks such as exits, becomes more realistic if not all conflicts are resolved immediately. Both these psychological and physical frictions lead to effects such as the formation of “arches” of the type seen in granular materials. These structures look similar to the barrel vaults found in bridges and other self-supporting structures held together by friction.
Another psychological phenomenon often associated with emergency situations is “panic”. Usually we associate panic with selfish and aggressive actions, and a breakdown of social order that is contagious in large groups. However, safety engineers have reviewed hundreds of disasters and found that, in the vast majority of cases, such behaviour has played no – or almost no – role in the tragic events. Instead, the opposite is usually observed, with most people acting co-operatively and altruistically even under extreme conditions. This has led experts to conclude that “panic” is the most abused terminology besides “chaos”, with the term “crowd disaster” being a more appropriate characterization.
But what happens in a real crowd disaster? Obviously one cannot deliberately recreate such events, and although some empirical data do exist in the form of reports from survivors or even video footage, it is almost impossible to derive quantitative results from these. Some researchers have, however, carried out evacuation experiments with animals to study the influence of “panic”, in which mice and ants were exposed to external hazards – water and repelling liquids, respectively. The animals showed a clear tendency towards herding, i.e. a preference for one of the available exits – a behaviour also seen in humans. After all, we might follow others because we think that they know the best way out, and in a dangerous situation we can feel more secure around others.
Particle people
People in a crowd might like to think they choose their own paths, but it turns out that we can borrow theories of many-particle systems from physics to accurately describe how crowds move and flow. Although variants of fluid-dynamical theories have long been used to study pedestrian streams, most modern models are microscopic, i.e. they distinguish between individual people.
Currently two main classes of model are used. The first is a deterministic approach that deals with forces and continuous variables. The idea of a description in terms of forces is based on the observation that a moving person can lead others to deviate from a straight path. Seen from a physics point of view, this implies acceleration and therefore the action of some “social” force. For pedestrian motion, social forces are repulsive and reflect the personal space of an individual. At higher densities, however, physical forces also play a role. Conceptually, social forces are quite different from physical forces because Newton’s laws are not necessarily obeyed. In general, the third law is not satisfied – as can be illustrated dramatically in the extreme case of stalking; here even the sign of the forces is different!
The second class of model is a stochastic framework in which we make decisions about where to move with some probability, reflecting the fact that two people can behave very differently when in exactly the same situation. In this model, people are generally described as cellular automata existing in a 2D grid of cells. A natural space discretization is given by the space occupied by a pedestrian in a dense crowd. Assuming a maximum density of 6.25 people per square metre, this corresponds to an area of 40 × 40 cm2 per person. Personal space is realized through an exclusion principle that allows each cell to be occupied by no more than one person. Time is also discrete and a single step is usually identified as the typical reaction time of a pedestrian – about 0.3 s. The dynamics in these models are defined in terms of transition probabilities to neighbouring cells.
The so-called floor-field model is a cellular automaton that goes a step further in implementing the rules. The probabilities are dynamic and depend on both the current and past particle configuration. Implicitly, it is assumed that pedestrians leave a trace, or footprints, that others have a tendency to follow, albeit subconsciously. This idea is analogous to the process of “chemotaxis”, as used by ants and other insects for communication. They leave a chemical trace to guide other individuals to food sources. The general principle in both cases is that a motion in the direction of stronger “fields” (pheromones or footsteps) is preferred.
In the evacuation assistant described in the main text, part of the research involves finding a suitable combination of force-based and floor-field models. The force-based model gives a more accurate representation of the geometry of the building, while the floor-field model is much faster in simulations.
Assisting with an evacuation
These studies of pedestrian dynamics are vital in understanding and thus being able to model the movement of people in the predictive code of our Hermes evacuation assistant. This system, which is still being developed, will not only provide data on the distribution of people in a stadium and the availability of rescue routes, but will also predict how they will leave. The first step involves obtaining accurate information about the current state of the crowd using ticket sales and a camera system that automatically counts people, giving the distribution of people in the facility. The stadium’s safety and security management system then provides Hermes with up-to-date information about, for example, the availability of emergency exits, the condition of fire doors and whether any smoke alarms have gone off. This information is subsequently fed into an evacuation simulation based on our models. Since the simulations run faster than real time, we can forecast areas where high densities and clogging could occur with potentially tragic consequences. This information is then sent to firefighters, police and security services, who can decide whether to take evasive action such as redirecting pedestrian streams in order to avoid congestion. A prototype of the evacuation assistant is currently being developed and the first components have already been completed. The first test, hopefully under normal conditions and not an emergency, will be performed in the summer of 2011.

Although a dynamic system like an evacuation assistant can have an enormous impact on the safety of mass events, sometimes static solutions through appropriate design of the facilities are helpful. An unexpected suggestion to improve evacuations is to put columns in front of exits, which should help to reduce friction effects and prevent arching. This idea works, at least in theory, as long as the presence of the columns does not change the behaviour of the people leaving.
While models of pedestrian dynamics have now become quite realistic, a lot still remains to be done. Quantitative predictions are difficult because so far there is not enough reliable empirical data to calibrate the models. However, reliable qualitative predictions are possible. These are sufficient at least to predict bottlenecks, and so can be used for forecasting as part of the evacuation assistant. Commercial simulation programs for evacuations are already available, but they have been found to produce largely deviating results, even by a factor of two in the simplest scenarios. What makes our project unique is the close co-operation between experimentalists and modellers, and the physics perspective that allows us to identify analogies with physical theories that can be built on and adapted.
So next time you leave a stadium, or any other large and crowded building, rather than dwelling on defeat you could take a moment to imagine yourself as not a person but a particle.
At a glance: Crowd dynamics
- Understanding how people move in crowds is important for architects wishing to design stadiums from which spectators can leave as quickly and safely as possible
- Physicists can play a role in this effort because crowds are essentially many-particle systems that can be modelled using both force-based and statistical physics
- When designing exit routes from stadiums, a key issue is knowing how pedestrian flow at bottlenecks changes as the constriction narrows or gets wider
- Researchers in Germany are developing an “evacuation assistant” that feeds live crowd data into software based on pedestrian models to provide stadium managers with information that can help them to decide how best to empty the facility
More about: Crowd dynamics
- A Schadschneider, D Chowdhury and K Nishinari 2010 Stochastic Transport in Complex Systems: From Molecules to Vehicles (Elsevier, Amsterdam)
- A Schadschneider et al. 2009 Evacuation dynamics: empirical results, modeling and applications Encyclopedia of Complexity and System Science ed A R Meyers (Springer, Berlin)
- Hermes project
- Ped-Net pedestrian and evacuation-dynamics site




