A new twist in our understanding of how fibres hold together in yarn and other spun materials has been revealed by three physicists in the UK. Using statistical physics to study a problem previously contemplated in 1638 by none other than Galileo, the trio has modelled how yarn makes a transition from being a weak material that can be easily pulled apart, to a much stronger material that only breaks when its fibres snap.
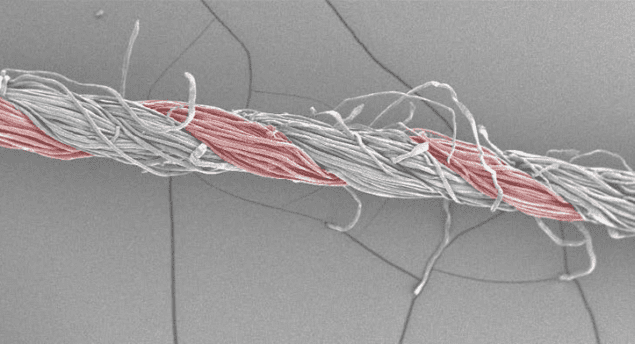
Few of us stop to think whether the spun and woven fibres that make our clothes will separate from one another, sparing no blushes as they fall in a heap on the floor. Although it is known that multiple frictional contact points between fibres hold clothes, ropes and yarn together, the mechanics behind why these fibre structures do not slip apart remains unclear.
Tension transfer
Patrick Warren at Unilever, Robin Ball at the University of Warwick and Raymond Goldstein of the University of Cambridge set out to answer this question by focussing on staple yarns. These are made of spun fibres, which are individually about 2-3 cm long and comprise wool, cotton or linen. The hypothesis of their study is that a yarn with few contact points between its fibres will fail by the fibres slipping past each other. However, they postulate, twisting the fibres together provides sufficient contact points so that normal forces acting between pairs of fibres allow tension to be transferred between them. This, they predict, should transform a weak yarn into a much stronger (up to 100-fold) material that can only fail by the fibres snapping.
To provide evidence for their hypothesis, the team translated the textile mechanics problem into an abstract linear programming problem. This involves treating the yarn as a collection of randomly overlapping near-parallel fibres and solving a system of linear inequalities representing the set of tensions in each of the fibre elements.
As a quantity representing the average tension transfer between fibres grew, the researchers witnessed a transition appear in their model, where the inequalities went from being unsatisfied to satisfied. In terms of the yarn, this represents the hypothesized transition from the fibres being free to slip, to a state in which the fibres are collectively locked together by friction, “The mechanical integrity reflects a genuine, and generic, phase transition in terms of a collective friction locking mechanism in fibre assemblies,” summarizes Goldstein.
Industry experience
Further statistical analysis revealed that this transition is smooth and depends on a combination of friction coefficient and turning angle per contact – where the turning angle is the number of twists in a fibre and a contact is a location where fibres are pinned together by friction. This provides a theoretical footing for what industry experience had shown – that yarn lubrication (which reduces the friction coefficient) and spinning (which increases the turning angle) are key “make or break” factors in the manufacturing process.

Researchers spin a yarn into a muscle
Although various approaches have been taken in the past that attempt to work-out how fibrous textile materials become so strong, Goldstein believes their statistical physics abstract model is “a novel application of linear programming, which could be transferrable to other situations involving static friction”. An important application could be the study of how stress is transmitted in granular materials – which could provide insight into everyday phenomena such as the behaviour of sand in piles and grain in silos.
Ning Pan of the University of California, Davis describes the research as a “fresh characterization” that applies new tools like percolation theory and linear programming to a long-standing problem. However, he also urges caution in how the work is interpreted “The abstract model is highly simplified and ‘unorthodox’, so it would be hard for future research to build on.”
The research is described in Physical Review Letters.