Imagine having an artificial substance in which you can control almost all aspects of the underlying periodic structure and the interactions between the atoms that make up this dream material. Such a substance would allow us to explore a whole range of fundamental phenomena that are extremely difficult – or impossible – to study in real materials. It may sound too good to be true, but over the last two years physicists have come extremely close to achieving this goal.

This breakthrough has been made possible by the convergence of two related but previously distinct realms of research in atomic physics: quantum gases and optical lattices. The new-found ability to confine ultracold quantum gases in optical lattices is already having a major impact in fields as diverse as condensed-matter physics and quantum information processing.

An optical lattice is essentially an artificial crystal of light – a periodic intensity pattern that is formed by the interference of two or more laser beams. The simplest optical lattice consists of the region of dark and bright stripes that is formed when two laser beams with the same wavelength travelling in opposite directions meet each other and form an interference pattern. This optical lattice has a period that is equal to half the laser wavelength. With more lasers – and enough care – it is possible to form a perfectly periodic 3D spatial structure. It is more difficult, however, to use these interference patterns to trap atoms.

An optical lattice is able to trap an atom because the electric fields of the lasers induce an electric dipole moment in the atom. The interaction between this dipole moment, which is oscillating, and the electric field of the laser modifies the energy of the atom. If the laser frequency is less than a specific electronic transition frequency within an atom, the atoms are pulled towards the regions of maximum laser intensity. However, if the laser frequency is higher than the transition frequency, the atoms are pushed away from the maxima. Either way the atoms can be trapped in the bright or dark regions of the optical lattice, and the strength of the optical potential confining the atoms can be increased by turning up the laser intensity (see Rolston in further reading).

Confining condensates

While some atomic physicists were experimenting with optical lattices, others were exploring what happens when atoms in magnetic traps are cooled so close to absolute zero that quantum statistics becomes important. The study of quantum gases has been one of the hottest – and coolest – areas of physics research since the first Bose-Einstein condensates were created in 1995, and remains so to this day (see “Fermionic first for condensates”). Over the past two years experimental groups in Europe and the US have learned how to transfer a quantum gas from a magnetic trap to an optical lattice, and this has led to a new generation of remarkable experiments.

The behaviour of a gas at temperatures close to absolute zero depends on whether the atoms in the gas are fermions or bosons. Fermions obey the Pauli exclusion principle, which means that two of them cannot occupy the same quantum state. No such restrictions apply to bosons, which means that large numbers of them can collapse into the same quantum ground state. This process – known as Bose-Einstein condensation – happens when bosonic atoms are cooled such that their de Broglie wavelength becomes comparable with the average distance between them.

In a Bose-Einstein condensate the de Broglie waves of the individual atoms “beat” in the same way, such that all the particles can be described by a single macroscopic wavefunction. Similar to laser radiation, condensates show a high degree of phase coherence. This means that if you know the phase of the matter wave at one point in space and time, you can predict what it will be at other points and times. Phase coherence is crucial for observing interference patterns.

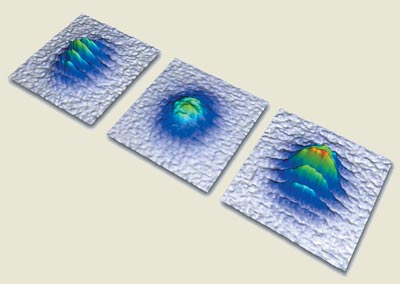
To transfer a Bose condensate from a magnetic trap to an optical lattice, the condensate is illuminated by the six laser beams needed to form a 3D lattice and the laser intensity is increased to the required value over a period of about 100 ms. This “loading” process is computer controlled to ensure perfect reproducibility between different experiments, but it is difficult to prove that the condensate has actually been transferred because the typical spacing between sites in the lattice is too small (about 430 nm) for the atoms to be imaged directly. However, a periodic pattern can show striking interference effects when illuminated with coherent waves, and we can use this approach to check that the condensate has indeed been transferred. We can also turn off the lattice potential and use the diffraction pattern to follow how the atoms on the different lattice sites expand and overlap. Since the wavefunctions in the condensate are phase coherent, an interference pattern builds up as they overlap (figure 1a).

Neutral atoms in optical lattices

In 1998 Peter Zoller of the University of Innsbruck in Austria and co-workers published an influential paper in which they suggested that it should be possible to convert a weakly interacting Bose gas into a Mott insulator – a strongly interacting quantum state – by loading the atoms into an optical lattice (see Jaksch et al. 1998 in further reading). Three years later, Ted Hänsch, the author and co-workers at the Max Planck Institute for Quantum Optics (MPQ) in Garching observed such a transition for the first time (see Greiner et al. in further reading). This publication is currently one of the most cited papers in physics according to the Science Watch newsletter.

But how does this change from a superfluid to an insulator actually come about? The potential depth in the optical lattice is almost always too large for the atoms to overcome the potential barrier between two neighbouring sites. On the other hand, quantum-mechanical tunnelling allows the atoms to spread through the lattice to some degree. In a Bose-Einstein condensate, this tunnelling process dominates the behaviour of the atoms, which causes the system to form a giant matter wave with perfect phase coherence between the matter waves on different lattices sites.

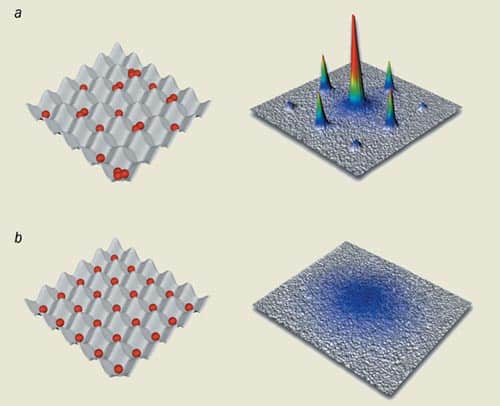
The number of atoms at each lattice site is a crucial factor in determining the properties of the system. If you tried to measure the number of atoms on each lattice site for a Bose-Einstein condensate, you would find that each site is filled with a random number of atoms according to Poisson statistics. In other words, the phase of the coherent matter wave is well defined but the number of atoms fluctuates from site to site. This is not due to technical imperfections, but rather a fundamental uncertainty in macroscopic quantum fields: whenever the phase of the macroscopic field is well defined, the number of atoms fluctuates and vice versa.

Although repulsive interactions between the neutral atoms are usually weak, they can be made to dominate the behaviour of the system by increasing the lattice depth and therefore reducing the amount of tunnelling. Placing two atoms at the same lattice site then becomes extremely costly in terms of energy, and the many-body system therefore tries to avoid doing it.

The best way to achieve this situation is to form a Mott insulator in which each lattice site is occupied by a single atom. The atoms are then isolated from each other, which results in the interaction energy being zero. In a Mott insulator the number of atoms on each lattice site is the same and does not fluctuate. However, this means that the phase coherence between atoms on neighbouring lattice sites has been completely lost, which makes it impossible to observe a matter-wave interference pattern (figure 1b).

In our experiments at MPQ we were able to convert a superfluid Bose-Einstein condensate to a Mott insulator and back again several times by controlling the depth of the optical lattice. One remarkable aspect about this phase transition is that it can occur even at a temperature of absolute zero. A classical system cannot change its configuration at zero temperature because the thermal fluctuations that drive such a transition are absent. For a quantum system, however, intrinsic vacuum fluctuations create a kind of quantum-mechanical “jitter”, which allows the system to change state even at absolute zero. Such quantum phase transitions are currently of great interest in condensed-matter physics and have also been observed in superconductors and quantum spin chains (see “Quantum criticality in metals”).

The quantum gases in our experiments were cooled to almost absolute zero, but they still had some finite temperature, and this allows defects to form in what would otherwise be a perfect Mott insulator. This is relevant to applications of optical lattices in, for example, quantum information: if the Mott insulator is to be used as a quantum register, then it is important that each site in the lattice contains the same number of atoms. We are also trying to find out which experimental parameters change most significantly at the transition to a Mott insulator, with the aim of gaining a more profound understanding of the phase transition itself.

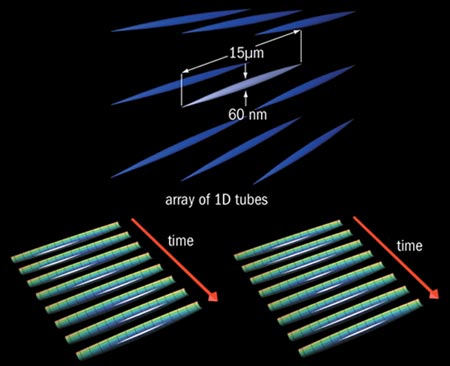
Optical lattices have also been used to investigate various intriguing aspects of 1D quantum gases. The dimensionality of a macroscopic quantum system can have a large impact on its physical behaviour, and it is therefore crucial to understand the role and effects of reduced dimensionality. Until recently, very few clean experimental systems had been developed in which such dimensionality effects could be studied. Now, however, arrays of 1D quantum gases can be produced using 2D optical-lattice potentials. In these gases the atoms are confined in tubes such that their radial motion is completely fixed and they can only move along the axial direction of the tube.

1D quantum gases often behave counterintuitively. For instance, unlike a 3D gas, the interactions between the atoms become stronger as the density of the gas is reduced. Tilman Esslinger and colleagues at the ETH in Zürich have recently demonstrated that 1D quantum gases with extreme aspect ratios (of about 250:1) can indeed be created in the lab. The ETH team has compared the oscillation frequencies of an axial “sloshing” motion, known as the atomic dipole mode, to that of a “breathing” motion of the atomic cloud (figure 2). For a 1D quantum gas one expects the oscillation frequencies of the two modes to have a distinct ratio, which was indeed observed in the experiment (see Moritz et al. in further reading).

Meanwhile, William Phillips and co-workers at NIST in Gaithersburg, US, have studied the initial effects on 1D quantum gases as they become more strongly interacting – a process called fermionization. This striking effect is caused by the repulsive interactions between the particles, which tends to separate the atoms from one other along the axial direction. As a result, atoms cannot be located at the same position in space, which effectively mimics the Pauli exclusion principle. This allows rubidium-87 atoms, which are bosons, to behave in some respects as if they were fermions (see Laburthe Tolra et al. in further reading).

As 1D bosonic quantum gases become even more strongly interacting, it should be possible to enter the regime of a Tonks-Girardeau gas, in which the fermionization is even more pronounced. The detection of such a gas would provide a textbook example of how a system of strongly interacting bosons can essentially be described by a free-fermion picture, and it is currently one of the most challenging goals in the ultracold field.

Quantum simulators

In addition to displaying novel quantum phases of many-body states, Bose-Einstein condensates in optical lattices offer great opportunities for quantum information processing. Atoms in a Mott insulating state can be viewed as a natural quantum register, in which each quantum bit (qubit) is represented by a single atom. The Mott transition can therefore be used to initialize a large set of qubits (up to 100,000) in a single experimental step. The challenge is to construct quantum logic gates between atoms that are trapped on different lattice sites (figure 3).

In 1999 Dieter Jaksch, now at Oxford University, Ignacio Cirac, now at MPQ, Peter Zoller and co-workers suggested an ingenious way to do this (see further reading). They proposed that state-dependent optical potentials could be used to bring neighbouring atoms together on a single lattice site. The idea was that both atoms would undergo a collision that would result in a precisely defined phase shift of the two-particle state. By setting this collisional phase shift equal to π, the system would constitute a quantum phase gate.

You might think that using atomic collisions for the delicate quantum control of two particles is not such a good idea, since collisions usually destroy the fragile coherence properties of quantum objects. However, collisions in the ultracold temperature regime are almost always fully coherent and therefore do not destroy the underlying quantum coherence. In the experiments one exploits the fact that atoms have an internal structure and can therefore exist in different internal states. In our experiments at MPQ these states correspond to different hyperfine states of the atom. To create spin-dependent potentials we use two counter-propagating lasers with polarizations that can be rotated relative to one another in a few microseconds. By exploiting this control over the beams, both internal states of the atom can experience different lattice potentials such that the atoms can be moved relative to one another.

If some atoms in the lattice that are initially in, say, a red internal state are converted into atoms in a blue state, these blue atoms can now be moved relative to the red atoms. Furthermore, they can be brought into contact with other red atoms in the lattice by moving the blue lattice potentials relative to the red lattice potential. By controlling such lattice shifts it is possible to move the atoms over a precisely defined separation and to bring them into contact with very distant neighbouring atoms.

This has allowed an exquisite degree of control over atoms in an optical lattice. For the first time we can completely control the interaction between two atoms that would otherwise never have interacted. Moreover, the periodic structure of the potential that the atoms experience has a powerful “parallelism” built into it: with just a single lattice-shift operation one can bring each atom into contact with its neighbouring atom, thereby forming a powerful quantum-gate array. Such quantum-gate arrays could be highly useful for generating entangled many-body states, which are a fundamental resource for quantum information processing (figure 4).

A major challenge in using optical lattices for quantum information processing is to address single atoms on different lattice sites. This would allow us to read and write information into selected atoms in the same way that an individual bit is used in a classical computer register. Recently Dieter Meschede and co-workers at the University of Bonn in Germany have shown that magnetic fields can be used to select single atoms that are separated by a few lattice sites, although it remains to be seen whether such an advanced level of control can be extended to the quantum register of a Mott insulator.

Phillips and co-workers at NIST are exploring other ways to address single atoms. In one experiment they have loaded atoms into every third site in the lattice by superimposing a so-called superlattice on top of a regular lattice pattern (see figure 5 and Peil et al. in further reading). The increased distance between the atoms should make it possible to use a focused laser to manipulate single atoms. The same team has also changed the spacing of the lattice by controlling the angle under which the laser beams interfere. In such an “accordion lattice” one could quickly change from a small lattice spacing to a large lattice spacing to address the atoms in the lattice.

Ultracold fermions in optical lattices

Spectacular advances in the field of degenerate Fermi gases now offer the possibility of loading ultracold fermionic gases into optical lattices. Last year, for instance, Massimo Inguscio and co-workers at the University of Florence in Italy studied the peculiar transport properties of bosons and fermions in periodic potentials. Here the cold fermionic quantum gas was slightly displaced from the optical trapping potential. The Florence team showed that the non-interacting fermions cannot support a DC current, which is what is expected given their special collisional properties and leads to a pinning of the atoms to their local displaced position. However, by adding a slight admixture of bosonic particles, the fermionic atom cloud relaxed towards the centre of the trap due to collisions between the bosons and the fermions.

Meanwhile, Misha Lukhin and colleagues at Harvard University have pointed out that degenerate Fermi gases in higher-dimensional optical lattices could be used to investigate high-temperature superfluidity. This could shed light on whether or not high-temperature superconductivity is contained within the “Hubbard model” that describes how interacting fermions behave in period potentials.

Unique systems

Ultracold atoms in optical lattices have the potential to impact on a broad range of physics. Not only can they be used as efficient quantum simulators for problems in condensed-matter physics, but it seems that the unique level of control that is available in these systems will also ensure their place in atomic physics and quantum optics. The huge quantum register provided by a Mott insulator, for example, offers unique possibilities for quantum information processing, although we still need to develop ways in which to address single atoms.

Another major challenge remains to engineer new many-body quantum phases, and to combine optical lattices with the recently observed condensation of molecules made from fermionic atom pairs. This special combination could allow us to explore some of the most fundamental many-body systems in condensed-matter physics. Our understanding of and ability to control quantum systems have never been so good.