A team of researchers in Spain says that it has developed an equation that describes how intricate surface patterns, resembling a cauliflower-like motif, evolve and develop over time. The researchers also show that their theory can be applied to everything from actual cauliflower plants to combustion fronts, all of which obey the same scaling laws. The team says that it is the first time that a theoretical explanation has been provided for the growth of surfaces in systems that are extremely dissimilar, be it their physical nature or the scale at which they grow.
The researchers based their study, published in New Journal of Physics, on two central tenets: fractals and universality. A fractal is an object or a quantity that is self-similar, or almost so, on all scales. The object need not exhibit exactly the same structure at all scales, but the same “type” of structures must appear on all scales. With a cauliflower, for example, it is impossible to tell if a close-up image of it is the entire head of the cauliflower or just a single floret. Simply put, a fractal is a system where any one part is similar to the whole.
Similar systems
Universality, on the other hand, refers to any physical systems that “look” extremely similar despite their specific details or the scale at which their effects are felt being very different. And fractals are a great example of universality – everything from a single fern leaf that resembles the entire plant, to clouds, snowflakes, blood vessels and cauliflowers has a similar fractal pattern. But despite the properties of fractals, such as their shapes and sizes, having been studied extensively since the 1970s, the physical mechanics of their formation have remained elusive.
Nanocauliflowers
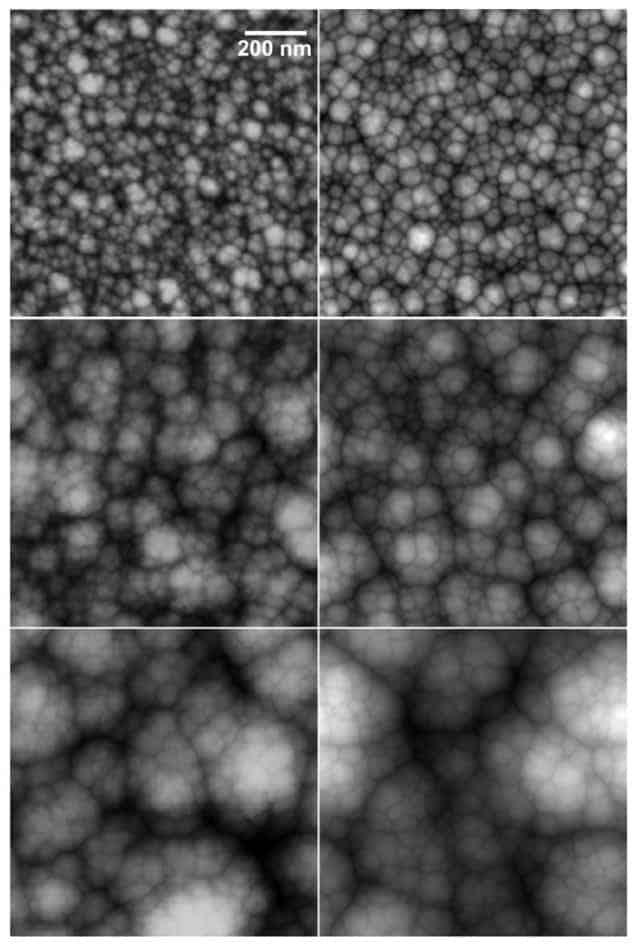
The new work has been carried out by Mario Castro and colleagues from Comillas Pontifical University, Universidad Carlos III de Madrid, Instituto de Ciencia de Materiales-CSIC, Ecole Polytechnique and Katholieke Universiteit Leuven. Initially, the researchers did not start out looking at fractals, instead they were studying a widely used technique to grow thin films known as chemical vapour deposition (CVD), which allows the thickness and composition of layers to be accurately controlled. The team was looking at the evolution of various films grown in the lab and found that one of the films – an amorphous hydrogenated carbon film – had an extremely recognizable if peculiar pattern, namely that of a cauliflower.
Inside joke
The team initially thought that its cauliflower motif was just that – a random pattern that had caught the eye. “It used to be our private joke almost – we used to call them our nanocauliflowers,” says Castro, who adds that although the films resemble cauliflowers, they are much smaller, being just several hundreds of nanometres. “But then we realized that our nanocauliflowers also had the same self-similar fractal features as those of the plant,” he adds.
Through experiments on its CVD carbon films, the team studied the cauliflower-like fronts, and developed a statistical formula that explains how these fronts grow over time. It turns out that this equation can also be used to successfully predict the growth of an actual cauliflower plant or even a combustion front – or how a flame grows over time – both of which occur at larger and different scales but obey the same scaling laws. “This proves our theory over seven orders of magnitude in length scales,” says Castro.
Ingredient’s list
The key finding in this work, according to the team, is the identification of the four ingredients needed for the formation of this kind of random growth. The first is a system that grows in time. This is crucial to the second part, which is “non-locality” or competition – a growing system, such as a plant trying to grow taller to receive more sunlight or even part of a flame expanding in time and trying to reach oxygen to combust. The non-locality plays a part here, as what happens in one part of the system remotely affects other distant parts of the same system. The third ingredient is randomness, which, according to the team, nature provides in abundance. The final ingredient is self-similarity – a fractal pattern, where the parts are similar to the whole.
“In spite of the widespread success of fractal geometry to describe natural and artificial fractal shapes, purely geometrical descriptions do not provide insight into the laws that govern the emergence of the shapes in time,” Castro told physicsworld.com. “We believe that by knowing the general laws that dictate how these patterns form and grow, it will help to identify the biological and physical mechanisms that are at play.” The team hopes its work will inspire other researchers to look into the real-world dynamics of fractals, instead of only their morphology.
The work is published in the New Journal of Physics.