Applying a magnetic field to graphene superlattices produces room temperature quantum oscillations created by delocalized quasiparticles known as magnetic Bloch states. These states should be generic to any superlattice system, not just graphene, and the new finding will be important for fundamental electron transport studies. It will also be crucial for characterizing and understanding novel superlattice devices based on 2D materials like graphene.
“Our study proves that it is possible to create new metallic systems within the same material by applying a magnetic field,” explains team member Roshan Krishna Kumar of the University of Manchester, UK. “These new metals have unique properties compared to those to which no magnetic field is applied, thus providing a new playground for studying condensed matter physics.”
New class of metallic system
The new result comes hot on the heels of work done last year, in which the researchers, led by Sir Andre Geim, observed robust (Brown-Zak) quantum oscillations in the magneto-conductivity of graphene (a sheet of carbon just one atom thick) at certain values of magnetic field. This study, published in Science DOI: 10.1126/science.aal3357, showed that these magnetic Bloch states, could be observed well above room temperature. The new work, detailed in PNAS this time, reveals high-order magnetic Bloch states that represent a new class of metallic system.
“Usually such experiments are done at liquid helium temperatures (around 4 K) to reduce lattice vibrations and study quantized phenomena,” says Krishna Kumar. “The data obtained can be rather difficult to interpret, however, because there can be multiple effects happening all at once. In graphene superlattices, for example, Brown-Zak and Shubnikov-de Haas oscillations co-exist at low temperatures. Our new approach involves heating samples to 100-200 K. This ‘smears’ most of the quantized effects so that our measurements only capture the behaviour of magnetic Bloch states.”
Fractal pattern in the magneto-conductivity
As reported in the PNAS paper, these states are present in second-, third-, and fourth-orders, and this full hierarchy creates a fascinating fractal pattern in the magneto-conductivity, Krishna Kumar tells nanotechweb.org. The pattern is intimately related to the way Hofstadter butterflies (striking fractal patterns that describe the behaviour of electrons in a magnetic field) originate. This complete fractal structure can indeed only appear due to high-order magnetic Bloch states and had never been seen in a graphene superlattice experiment until now.
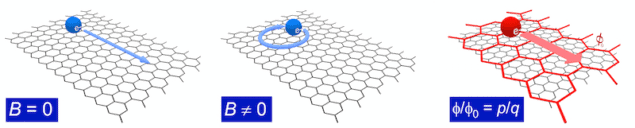
A Bloch state is the quantum mechanical description of an electron in a solid-state crystal. It explains one of the puzzling questions in condensed matter physics: how can a negatively charged electron move through the crystal without bumping into the positively-charged cores?
Electrons carry on moving as if there were no field
“If we apply a strong magnetic field perpendicular to the crystal, we destroy this Bloch state because electrons experience the Lorentz force and become ‘stuck’ on closed orbits,” explains Krishna Kumar. “In our experiments, we show that electrons can move freely as a Bloch state under a certain applied magnetic field (one whose ‘magnetic length’ is commensurable with the periodicity of the superlattice). In other words, the electrons carry on moving as if there were no field applied.”
Mathematically, this effect occurs each time an integer number of magnetic flux quanta (φ) pierce an integer number of crystal unit cells, according to φ = SB = φ0p/q, where S is the area of a crystal’s unit cell and p and q are integer numbers. “It is impossible to find such magnetic Bloch states in typical crystals because we would require unfeasibly high magnetic fields (of 10 000 Tesla) to see them, says Krishna Kumar. However, the beauty of graphene superlattices is that they are significantly larger than naturally occurring crystals (50 times larger in our case). In these systems, we only have to apply field of around 4 Tesla to observe the magnetic Bloch states, which is relatively easy with the magnets available today.
Any superlattice
We should be able to observe these Bloch states in any superlattice, not just graphene, he adds. “Our findings will be important for electron transport studies, since the physics involved is one of the most fundamental aspects of magneto-transport in solid state-crystals. Our work will thus help us better characterize and understand novel devices based on 2D superlattice materials.
“These states are essentially a new class of material with different properties compared to ordinary graphene. For example, many of the first-order magnetic Bloch states host pronounced band gaps, in contrast to graphene, which is a zero-gapped semi-metal. Now that we have identified higher-order states, our next step will be to understand exactly how they behave.



